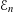Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ching, Michael
and
Salvatore, Paolo
2022.
Koszul duality for topological
E
n
‐operads
.
Proceedings of the London Mathematical Society,
Vol. 125,
Issue. 1,
p.
1.
Zhang, Yu
2022.
Homotopy pro-nilpotent structured ring spectra and topological Quillen localization.
Journal of Homotopy and Related Structures,
Vol. 17,
Issue. 4,
p.
511.
Bayindir, Haldun Özgör
and
Péroux, Maximilien
2023.
Spanier–Whitehead duality for topological coHochschild homology.
Journal of the London Mathematical Society,
Vol. 107,
Issue. 5,
p.
1780.
Malin, Connor
2024.
Koszul self‐duality of manifolds.
Journal of Topology,
Vol. 17,
Issue. 2,