Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T18:48:43.251Z Has data issue: false hasContentIssue false
  • English
  • Français

Amplification of filamentation instability by negative hydrogen ions stream driven by a magnetized counterstreaming e–H− plasmas

Published online by Cambridge University Press:  10 June 2015

Mohammad Ghorbanalilu  and
Babak Shokri
Mohammad Ghorbanalilu*
Affiliation:
Physics Department, Shahid Beheshti University, G.C., Tehran, Iran
Babak Shokri
Affiliation:
Physics Department, Shahid Beheshti University, G.C., Tehran, Iran
*
Address correspondence and reprint requests to: Mohammad Ghorbanalilu, Physics Department, Shahid Beheshti University, Evin, Tehran, Iran. E mails: mh_alilo@yahoo.com and m_alilu@sbu.ac.ir
Get access Rights & Permissions [Opens in a new window]

Abstract

The main purpose of this theory is to present a simple picture of magnetic field generation by a relativistic equilibrium counterstreaming electron–negative hydrogen ion (e–H−) plasmas propagating parallel to an ambient external magnetic field. The existence of such kind of plasma flows can be imagined during the negative hydrogen ion propagation through neutralizing plasma, in order to generate an energetic neutral hydrogen beam. The produced magnetic field deflects the electron and negative hydrogen ion flows and reduces the efficiency of hydrogen neutral beam generation. We focused our analysis on the influences of the negative hydrogen ion contribution, the particles thermal velocity and the external magnetic field on the growth rate of generated sheared magnetic field. The dispersion relation is obtained using a relativistic two-fluid model and Maxwell equations. The analytical and numerical solutions admit generation of a purely growing transverse electromagnetic field across the ambient external magnetic field. It is shown that H− current filaments are responsible for deep penetration of the sheared magnetic fields into plasma, however, applying a weak magnetic field ${\rm \omega} _{{\rm ce}}^2 {\rm \ll} {\rm \omega} _{{\rm pe}}^2 $ suppresses magnetic field generation for a counterstreaming e–H− plasma in the absence of H− ions dynamics. On the other hand, a magnetic field exists with a small growth rate for strongly magnetized (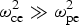${\rm \omega} _{{\rm ce}}^2 \;{\rm \gg}\; {\rm \omega} _{{\rm pe}}^2 $) e–H− plasma when the influence of H− ions is included. Although the growth rate is small, we expect that magnetic field generation is further amplified and the penetration depth is increased owing to H− ions stream, on a time scale much longer than the plasma period $t\;{\rm \gg}\; {\rm \omega} _{{\rm pe}}^{ - 1} $.

Keywords

Electron beamsFilamentation instabilityMagnetized plasmaTwo-fluid model
Type
Research Article
Information
Laser and Particle Beams , Volume 33 , Issue 3 , September 2015 , pp. 481 - 487
Copyright
Copyright © Cambridge University Press 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abraham-Shrauner, B. (2010). Weibel instability and quasi-equilibria for collisionless plasmas. Plasma Phys. Control Fusion 52, 025003.CrossRefGoogle Scholar
Allen, B., Yakimenko, V., Babzien, M., Fedurin, M., Kusche, K., Muggli, P. (2012). Experimental study of current filamentation instability. Phys. Rev. Lett. 109, 185007.CrossRefGoogle ScholarPubMed
Ardaneh, K., Cai, D. & Nishikawa, K.H. (2014). Amplification of Weibel instability in the relativistic beam plasma interactions due to ion streaming. New Astron. 33, 1–6.CrossRefGoogle Scholar
Bendib, K., Bendib, A., Bendib, K., Bendib, A., Sid, A. & Bendib, K. (1998). Weibel instability analysis in laser-produced plasmas. Laser Part. Beams 16, 473.CrossRefGoogle Scholar
Bret, A. (2013). Robustness of the filamentation instability for asymmetric plasma shells collision in arbitrarily oriented magnetic field. Phys. Plasmas 20, 104503.CrossRefGoogle Scholar
Bret, A. (2014). Robustness of the filamentation instability in arbitrarily oriented magnetic field: Full three dimensional calculation. Phys. Plasmas 21, 022106.CrossRefGoogle Scholar
Bret, A., Christine Firpo, M. & Deutsch, C. (2005). Bridging the gap between two stream and filamentation instabilities. Laser Part. Beams 23, 375.CrossRefGoogle Scholar
Bret, A., Christine Firpo, M. & Deutsch, C. (2006). Between two stream and filamentation instabilities: Temperature and collisions effects. Laser Part. Beams 24, 27.CrossRefGoogle Scholar
Bret, A., Gremillet, L. & Bellido, J.C. (2007). How really transverse is the filamentation instability? Phys. Plasmas 14, 032103.CrossRefGoogle Scholar
Brian Yang, T.-Y., Gallant, Y., Arons, J. & Bruce, A. (1993). Weibel instability in relativistically hot magnetized electron–positron plasmas. Phys. Fluids B 5, 3369.CrossRefGoogle Scholar
Califano, F., Attico, N., Pegoraro, F., Bertin, G. & Bulanov, S.V. (2001). Fast formation of magnetic islands in a plasma in the presence of counterstreaming electrons. Phys. Rev. Lett. 86, 5293.CrossRefGoogle Scholar
Califano, F., Del Sarto, D. & Pegoraro, F. (2006). Three-dimensional magnetic structures generated by the development of the filamentation (Weibel) instability in the relativistic regime. Phys. Rev. Lett. 96, 105008.CrossRefGoogle ScholarPubMed
Fiore, M., Silva, L.O., Ren, C., Tzoufras, M.A. & Mori, W.B. (2006). Baryon loading and the Weibel instability in gamma-ray bursts. Mon. Not. R. Astron. Soc. 372, 1851.CrossRefGoogle Scholar
Fonseca, R.A., Silva, L.O., Tonge, J.W., Mori, W.B. & Dawson, J.M. (2003). Three-dimensional Weibel instability in astrophysical scenarios. Phys. Plasmas 10, 1979.CrossRefGoogle Scholar
Fried, B. (1959). Mechanism for instability of transverse plasma waves. Phys. Fluids 2, 337.CrossRefGoogle Scholar
Ghorbanalilu, M. (2006). The Weibel instability on strongly magnetized microwave produced plasma. Phys. Plasmas 13, 102110.CrossRefGoogle Scholar
Ghorbanalilu, M. (2011). On the stability analysis of electromagnetic waves along the external magnetic field in a magnetized microwave-produced plasma. Plasma Phys. Control Fusion 53, 035006.CrossRefGoogle Scholar
Ghorbanalilu, M. (2013). Resonance and non-resonance Weibel-like modes generation in optical breakdown of a dilute neutral gas by an intense laser field. Plasma Phys. Control Fusion 55, 045002.CrossRefGoogle Scholar
Ghorbanalilu, M., Sadegzadeh, S., Ghaderi, Z. & Niknam, A.R. (2014). Weibel instability for a streaming electron, counterstreaming e–e, and e–p plasmas with intrinsic temperature anisotropy. Phys. Plasmas 21, 052102.CrossRefGoogle Scholar
Hao, B., Sheng, Z.-M. & Zhang, J. (2008). Kinetic theory on the current-filamentation instability in collisional plasmas. Phys. Plasmas 15, 082112.CrossRefGoogle Scholar
Hao, B., Sheng, Z.-M., Ren, C. & Zhang, J. (2009). Relativistic collisional current-filamentation instability and two-stream instability in dense plasma. Phys. Rev. E 79, 046409.CrossRefGoogle ScholarPubMed
Kennel, C.F. & Petschek, H.E. (1967). Collisionless shock waves in high β plasmas: 1. J. Geophys. Res. 72, 3303.CrossRefGoogle Scholar
Lazar, M. (2008). Fast magnetization in counterstreaming plasmas with temperature anisotropies. Phys. Lett. A 372, 2446.CrossRefGoogle Scholar
Lazar, M., Dieckmann, M.E. & Poedts, S. (2010). Resonant Weibel instability in counterstreaming plasmas with temperature anisotropies. J. Plasma Phys. 76, 49.CrossRefGoogle Scholar
Liu, Y.-H., Swisdak, M. & Drake, J.F. (2009). The Weibel instability inside the electron–positron Harris sheet. Phys. Plasmas 16, 042101.CrossRefGoogle Scholar
Okada, T., Sajiki, A. & Satou, K. (1999). Weibel instability by ultraintense laser pulses. Laser Part. Beams 17, 515.CrossRefGoogle Scholar
Pegoraro, F., Bulanov, S.V., Califano, F. & Lontano, M. (1996). Nonlinear development of the weibel instability and magnetic field generation in collisionless plasmas. Phys. Scr. T 63, 262.CrossRefGoogle Scholar
Quinn, K., Romagnani, L., Ramakrishna, B., Sarri, G., Dieckmann, M.E., Wilson, P.A., Fuchs, J., Lancia, L., Pipahl, A., Toncian, T., Willi, O., Clarke, R.J., Notley, M., Macchi, A. & Borghesi, M. (2012). Weibel-induced filamentation during an ultrafast laser-driven plasma expansion. Phys. Rev. Lett. 108, 135001.CrossRefGoogle ScholarPubMed
Sakai, J., Schlickeiser, R. & Shukla, P.K. (2004). Simulation studies of the magnetic field generation in cosmological plasmas. Phys. Lett. A 330, 384.CrossRefGoogle Scholar
Schlickeiser, R. & Shukla, P.K. (2003). Cosmological magnetic field generation by the Weibel instability. Astrophys. J. 599 L57, 1538.Google Scholar
Shokri, B. & Ghorbanalilu, M. (2004 a). Relativistic effects on the Weibel instability of circularly polarized microwave produced plasmas. Phys. Plasmas 11, 5398.CrossRefGoogle Scholar
Shokri, B. & Ghorbanalilu, M. (2004 b). Wiebel instability of microwave gas discharge in strong linear and circular pulsed fields. Phys. Plasmas 11, 2989.CrossRefGoogle Scholar
Shukla, N. & Shukla, P.K. (2007). Generation of magnetic field fluctuations in relativistic electron–positron magnetoplasmas. Phys. Lett. A 362, 221–224.CrossRefGoogle Scholar
Tautz, R.C. & Sakai, J.-I. (2007). Magnetic field amplification in anisotropic counterstreaming pair plasmas. Phys. Plasmas 14, 012104.CrossRefGoogle Scholar
Tzoufras, M., Ren, C., Tsung, F.S., Tonge, J.W., Mori, W.B., Fiore, M., Fonseca, R.A. & Silva, L.O. (2006). Space-charge effects in the current-filamentation or Weibel instability. Phys. Rev. Lett. 96, 105002.CrossRefGoogle ScholarPubMed
Weibel, E.S. (1959). Spontaneously growing transverse waves in a plasma due to an anisotropic velocity distribution. Phys. Rev. Lett. 2, 83.CrossRefGoogle Scholar
Yalinewich, A. & Gedalin, M. (2010). Instabilities of relativistic counterstreaming proton beams in the presence of a thermal electron background. Phys. Plasmas 17, 062101.CrossRefGoogle Scholar