1. Introduction
The energy loss of charged particle in matter has been devoted a large number of investigations, both theoretical and experimental [Reference Olsen, Mehlhorn, Maenchen and Johnson1–Reference Gardes, Bimbot and Rivet7], in which the interaction of ion beams with cold matter has already obtained a plausible understanding, and theoretical predictions are in a good agreement with the experimental data [Reference Paul8]. Plasma, however, as a fundamental state of matter in our Universe, is only poorly understood and lacks reliable experimental data testing. In plasma physics, the basic problem is the interaction and energy loss of ion beams in the plasma [Reference Spitzer9–Reference Li and Petrasso12]. In this subject, the energy loss of kinetic ions in plasma is very important for the development of inertial confinement fusion (ICF) [Reference Nuckolls, Wood, Thiessen and Zimmerman13, Reference Kawata, Karino and Ogoyski14], ion-driven fast ignition [Reference Tabak, Hammer and Glinsky15, Reference Roth, Cowan and Key16], and high-energy-density physics (HEDP) investigation [Reference Sharkov, Hoffmann, Golubev and Zhao17]. Meanwhile, it has many practical applications in medicine, material science, accelerator technology, and so on.
The energy loss of ions in a neutral gas is dominated by the collisions with bound electrons, while the energy loss in plasma is deduced to the collisions with free electrons.. In cold gaseous target, the van der Waals collision is dominating, while in plasma the electrostatic interaction becomes important [Reference Shukla and Akbari-Moghanjoughi18]. A large amount of experimental data for the stopping of ions in cold matter has been accumulated, where the inelastic collision between ions and bound electrons plays a leading role [Reference Ahlen19]. However, only few experimental data are available for the stopping of ions in plasmas, in which the collision between ions and free electrons is prevailing and the enhancement of the Coulomb energy losses is observed.
In principle, two main terms are found to increase the stopping of ions in plasma: one is the increase of Coulomb logarithm due to high-frequency energy transfer between ions and free electrons, and the other is the increased effective charge of projectiles in the plasma. The charge state of a projectile moving in the plasma is determined by the dynamic equilibrium of ionization and recombination. The charge state is expected to be higher compared to cold gas, and the reason could be deduced from the reduced cross section of direct capture of a free electron compared to that of a bound electron [Reference Nardi and Zinamon20–Reference Peter, Arnold and Meyer-ter-Vehn22]. In plasma, the energy loss of ions becomes larger with the increase of charge state. At present, the effective charge theory of energy loss has become a powerful tool to correlate the experimental data [Reference Dietrich, Hoffmann and Boggasch6, Reference Nardi and Zinamon20].
In general, the energy loss of heavy ions can be extrapolated simply from the energy loss of protons in the same material and with the same velocity, in which they can be correlated by the effective charge of ions [Reference Gus’kov, Zmitrenko, Il’in, Levkovskii, Rozanov and Sherman23]. Moreover, the energy loss of protons is also very important for nuclear fusion and ion-driven fast ignition. In order to investigate the effective charge state of heavy ions in beam-plasma interaction, the protons can make a comparative measurement of energy loss [Reference Golubev, Basko and Fertman24, Reference KulishFortov, Gryaznov and Kulish25]. Meanwhile, the energy losses of protons can be used as a practical diagnostic method to measure the density of free electrons in plasmas [Reference Golubev, Turtikov and Fertman26]. In application, P-11B reaction provides a new solution for ignition, in which the resonant energy is about the magnitude of hundred keV [Reference He, Jia and Xu27, Reference Putvinski, Ryutov and Yushmanov28]. Thus, the energy loss of proton with the energy of hundred keV in plasma is an important topic for fusion development. It is proposed that the use of diatomic molecular ions and cluster ion beams of hydrogen may also prove helpful to drive inertial confinement fusion [Reference Wang, Yi and Li29]. In addition, collective effect of protons in dense plasma has been investigated, and it is essential for the design of ion-driven fast ignition and inertial confinement fusion [Reference Ren, Deng and Qi30]. In the low-energy regime, the energy-loss measurement for 100 keV proton in the hydrogen plasma has been presented in our previous work [Reference Cheng, Zhou and Wang31], and the energy-loss enhancement effect is attributed to the higher Coulomb logarithm. Nevertheless, the effective charge for protons in the plasma is thought as 1, i.e., the charge-state evolution is not mentioned. In [Reference Zhang, Liu, Cheng, Zhao and He32], the effective charge for 100 keV proton in the hydrogen plasma has been calculated and discussed again. Moreover, the empirical formulae near the Bragg peak have been used to extract the effective charge [Reference Cayzac, Frank and Ortner33]. However, the energy loss of ions in plasma for the low-energy regime has not been completely clear yet due to the lack of experiments, and the theoretical predictions also consist of large uncertainties [Reference Gericke and Schlanges34, Reference Cayzac, Bagnoud and Basko35].
In this work, we present a new experimental data of energy losses of 90 keV and 100 keV protons penetrating through the hydrogen plasma, and the effective charge state in the low-energy regime is discussed through the empirical formula calculations.
2. Experimental Setup
The experiment was performed at the 320 kV high-voltage experimental platform at the Institute of Modern Physics, Chinese Academy of Sciences (IMP, CAS), Lanzhou [Reference Cheng, Zhou, Sun, Lei, Wang and Xu36, Reference Zhao, Hu and Cheng37]. Proton beams were extracted from the electron cyclotron resonance (ECR) ion source and selected by two 90° bending magnets. The protons were accelerated to 90 and 100 keV, respectively, and then introduced into a special experimental terminal for ions and plasma interaction investigation. The experimental system has been fully described in the previous work [Reference Cheng, Zhou and Wang31]. In brief, the proton beams with a spot size of about φ1 mm penetrated through the hydrogen plasma target. After through the plasma, a 0.5 m radius bending magnet with a deflection of 45° and a coupled time-resolved position-sensitive detector were used to measure the position of protons. The remained energy of the proton can be obtained from the position shift which is a function of the velocity of protons and magnetic field intensity. If the protons lose a certain amount of energy (dE) in the plasma, the position of the outgoing beam at the detector shifts by dx correspondingly. The range of the delay time (after the ignition of the discharge) of the detector is about 200 ns–20 ms, and the width of the detection time is from 10 ns up to infinity. The spatial resolution was about 70 μm. A good spatial resolution detector and the very stable magnetic field of the bending magnet were employed. The size of the proton beam was only 1 mm which is corresponding to 1 keV energy difference. In the experiment, the shift distance of the beam is about 0.5 mm. So the resolution of energy loss on the detector system is about 0.5 keV.
The plasma target based on a linear electric discharge in Z-pinch geometry was applied to study the energy loss of charged particles in ionized matter. The plasma will exist in about 8 μs and the temperature is about 1-2 eV. A Rogowski coil is used to measure the temporal discharge current (see Figure 3 in [Reference Cheng, Zhou and Wang31]) and the start pulse signal for triggering the detector is derived from the rising edge when the voltage is higher than 0.89 V. The higher discharge voltage produced larger current intensity and higher ionization degree, and free-electron density becomes larger. The maximum of the free-electron density is at about 3 μs (relative to the ignition) for the different discharge voltages. The plasma linear electron density has been determined by the laser interferometry techniques [Reference Kuznetsov, Byalkovskii and Gavrilin38]. The length of the gas column in the target is limited to about 220 mm. The vacuum system of the beam line is protected from the gas load of the target by means of differential pumping. For the initial pressure of the hydrogen gas ranging 1∼9 mbar, the free-electron density of 1016−17 cm−3 can be created in such a discharge.
3. Results and Discussion
A typical energy-loss measurement spectrum of 100 keV proton penetrating through the hydrogen plasma at different discharging time (the initial gas pressure was about 0.81 mbar, and the voltage was 3 kV) is shown in Figure 1. Figures 1(a) and 1(b) show the measured positions of ions in the detector at 0 μs and 3 μs time, respectively. The systematical energy loss can be obtained by measuring the position shift at different discharging time. Here, that energy loss increases by 4.07 keV comparing to the cold gas can be found in Figure 1.
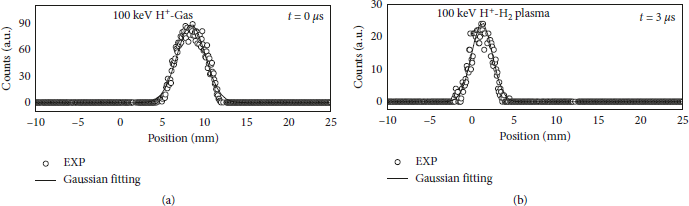
Figure 1: A typical energy-loss measurement spectrum of 100 keV proton penetrating through hydrogen gas discharging plasma at different discharging time (a) was at 0 μs time and (b) was at about 3 μs time (the initial gas pressure was about 0.81 mbar, and the voltage was 3 kV).
The plasma state has been diagnosed by Kuznetsov, and in our experiment, the plasma state can be determined by the initial gas pressure and discharge voltage based on the results presented in [Reference Kuznetsov, Byalkovskii and Gavrilin38]. The initial energy loss ΔE for 100 and 90 keV protons was measured to be 5.02 and 7.73 keV before discharge. The gas pressure is determined to be 0.81 mbar and 1.25 mbar according to the measured ΔE (see [Zhang et al., 2020]. for details). With the discharge voltage of 3 kV, the linear free-electron density n f and average ionization degree of the plasma are found to be 3.35∗1017 cm−2 and 3.75∗1017 cm−2, and 0.76 and 0.44, respectively, at the peak stage of discharge (around 3 μs). The linear free- and bound-electron density can be obtained by ref [Reference Kuznetsov, Byalkovskii and Gavrilin38]. Figure 2 shows the free- and bound-electron density (the initial gas pressure was about 0.81 mbar, and the voltage was 3 kV), where n b and n f denote the linear bound- and free-electron density, respectively. n f gradually rises up until the onset of the discharge peak stage. Then, it gradually decreases with the discharge time. Meanwhile, n b evolves in the opposite tendency.

Figure 2: Linear free-electron (solid line) and bound-electron densities in plasma as a function of discharge time (the initial gas pressure was about 0.81 mbar, and the voltage was 3 kV).
The energy-loss change in the whole plasma lifetime was recorded as a function of time after the discharge, which is shown in Figure 3 where the theoretical prediction is also shown for comparison. In our experiment, the total uncertainly of the energy loss is about 10% mainly from the broadening of the ion beam spot and the detector itself. Figures 3(a) and 3(b) represent energy losses of 100 keV and 90 keV protons in plasma (the discharging voltage was 3 kV), respectively. The initial gas pressures were estimated to be 0.81 mbar for 100 keV proton incident and 1.25 mbar for 90 keV proton incident. It should be noted that a similar trend of the change of discharge current, free-electron density, and energy loss of protons as a function of time can be observed in [Reference Cheng, Zhou and Wang31]. Both the discharging current and energy loss are mainly dependent on the free-electron density in the plasma, and for the first 1 microsecond, the discharge current and energy loss are not very stable (see ref. [Reference Cheng, Zhou and Wang31] for details), which is probably due to the fast changing of the electromagnetic field in the beginning. It results in decrease of energy loss at the beginning of discharge (0-1 μs). The phenomena, however, have not been clear yet, and a similar case is also found in refs. [Reference Frank, Blažević and Grande39, Reference Frank, Blažević and Bagnoud40]. When the discharging current reaches the maximum at around 3 μs after discharging, the temporal gradients of the electromagnetic field and the plasma parameters are minimum. Thus, the energy loss reaches the maximum at around 3 μs where the plasma reaches its most stable state. We choose the experimental data from the relatively stable plasma state from 2 to 4 μs to carry out the discussion below.
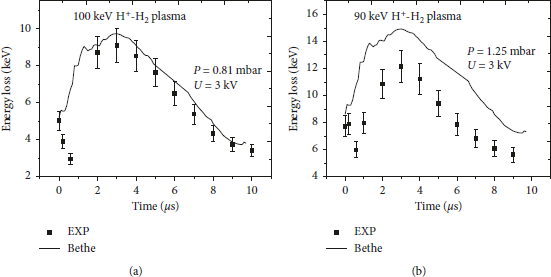
Figure 3: Energy losses of protons penetrating through hydrogen gas discharging plasma. (a) 100 keV proton in plasma (the initial gas pressure was about 0.81 mbar, and the voltage was 3 kV); (b) 90 keV proton in plasma (the initial gas pressure was about 1.25 mbar, and the voltage was 3 kV). The symbols represent experimental data. The solid line represents the theoretical predictions of the Bethe model with Z eff = 1.
In a partially ionized plasma target, the incident ions lose their energy through cascade collisions with free electrons and/or bound electrons. Considering the homogeneity of the plasma target and the (nearly zero) slope of the stopping power function at this energy regime, a stepwise integration is not necessary and the total energy loss ΔE can be expressed as follows:
where L = 15.6 cm is the plasma target length and [dE/dx]free and [dE/dx]bound represents the stopping power of the free electrons and bound electrons, respectively.
According to the Bethe model, the stopping power from the aspects of free electrons and bound electrons can be represented as follows:
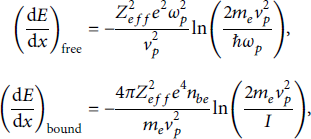
where
![]() is the plasma frequency, Z
eff is the projectile effective charge state, vp denotes the projectile velocity, m
e
and e are the electronic mass and charge, and n
be and n
fe are the density of free electrons and bound electrons, respectively, in which the degree of ionization has been considered. I = ħϖ is the average excitation energy of target atoms, which is 15 eV for hydrogen atom [Reference Belyaev, Basko and Cherkasov41].
is the plasma frequency, Z
eff is the projectile effective charge state, vp denotes the projectile velocity, m
e
and e are the electronic mass and charge, and n
be and n
fe are the density of free electrons and bound electrons, respectively, in which the degree of ionization has been considered. I = ħϖ is the average excitation energy of target atoms, which is 15 eV for hydrogen atom [Reference Belyaev, Basko and Cherkasov41].
In the present work, when Z eff is chosen as 1, the experimental data for 100 keV proton incident can be well reproduced by Bethe theoretical predictions, which is consistent with our previous results [Reference Cheng, Zhou and Wang31]. However, for 90 keV proton incident, the theoretical calculations obviously overestimate the experimental data by a factor of about 2, which may be attributed to the charge-state evolution of protons in the plasma [Reference Olson and Salop42].
In [Reference Olson and Salop42], the classical trajectory calculations were used to predict the charge-transfer and impact-ionization cross sections for collisions of H+-H in the velocity range of 2–7 × 108 cm/sec, which is equivalent to the ion velocity in our experiment. Here, the charge-exchange cross section corresponds to a capture into any of the bound states of the ions, that is, a total capture cross section rather than a capture into the ground state only. The projectile charge state is determined by the total electron-loss cross sections (sum of charge exchange and impact ionization). One can clearly see that impact-ionization cross sections for 100 keV and above 100 keV H+-H collisions are dominated. This means that, in this case, the charge-transfer cross sections can be ignored, in which the cross sections decrease with the increase of incident energy, while for below 100 keV H+-H collisions, the total electron-loss cross sections are determined by both the charge-transfer cross sections and the impact-ionization cross sections. Therefore, the charge-state evolution effect needs to be taken into account for below 100 keV H+-H collisions. Based on the discussion above, when Z eff is equal to 1, the theoretical predictions for 90 keV proton incident overestimate the experimental data, which can be explained by the charge-state evolution effect. The experimental phenomenon concerned with the variation of the effective charge of protons in the plasma has not been reported so far. In our experiment, for the low-energy regime, when the incident energy is 100 keV, the effective charge of protons is equal to 1. While for 90 keV proton incident, the charge-state evolution needs to be considered, Z eff should be less than 1 [Reference Olson and Salop42].
In the present work, the effective charge of 90 keV protons in the hydrogen plasma can be calculated through some empirical formulae. Kreussler et al. [Reference Kreussler, Varelas and Brandt43] suggested that the equilibrium charge state of projectile ions can be used to estimate the energy loss. The equilibrium charge state relies on the relative velocity of the projectile v to the electrons of the target ve, in which all the possible orientations of vector v − ve are considered, which is given by

In the case of plasma, the electron velocity is determined by its corresponding Fermi velocity and the thermal velocity of free electrons:
where T is the plasma temperature, E F is Fermi energy, and k B is the Boltzman constant. The effective charge state is then calculated by
where Z is the projectile atomic number.
Moreover, Gus’kov et al. [Reference Gus’kov, Zmitrenko, Il’in, Levkovskii, Rozanov and Sherman23] proposed a similar model, in which the effective charge is defined by the following relationship:
Here, the typical parameter is given by
In the Kreussler model, Z eff is equal to 0.861. In the Gus’kov model, the value of Z eff is 0.832. Figure 4 shows the theoretical calculations from two empirical models, in which they all underestimate the experimental data. The main reason is that the parameters of only incident ions in two empirical formulae are considered, while target properties are ignored. In our experiment, the plasma is partially ionized, and ionization degree should be taken into account [Reference Zhang, Liu, Cheng, Zhao and He32].
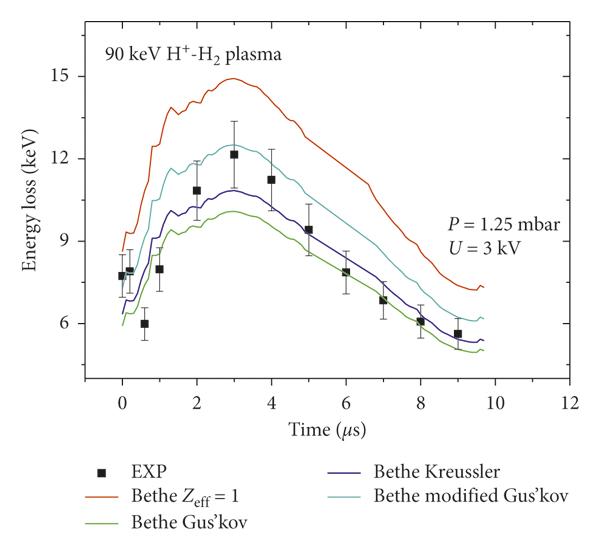
Figure 4: Energy losses of 90 keV protons in hydrogen gas discharging plasma. Theoretical model calculations are also shown for comparison.
Compared to neutral matter, plasmas have different components (atoms, ions, and electrons), and the component density depends on plasma temperature and density. In order to describe the interaction between incident ions and plasma, the rate constants of the processes are employed, i.e., N〈υ σ〉(s−1) [Reference Tolstikhina and Shevelko44]. The constant quantities averaged over a Maxwellian distribution of particle velocities v; here, N is the particle density of the plasma. Therefore, in the present work, the ionization degree of plasma needs to be considered for the calculation of the effective charge of the projectile. Moreover, for the empirical formulae, it is necessary that the mean values of the relative velocity and fluctuations of the quantities also need to be taken into account [Reference Gus’kov, Zmitrenko, Il’in, Levkovskii, Rozanov and Sherman23].
In this work, the value of Z eff is found to be about 0.92, and the theoretical predictions give a good description with the experimental data, as shown in Figure 4. In [Reference Ziegler, Biersack and Littmark45, Reference Brown and Moak46], to achieve better agreement with the experimental data, various typical parameters of expression (5) have been used. Here, expression (5) can be modified as follows:
Thus, our experimental results can be reproduced by the theoretical predictions.
Similar calculations for 100 keV protons are also applied. Basing on the Gus’kov and Kreussler models, the values of Z eff are 0.846 and 0.864, respectively. They all underestimated the experimental data, as shown in Figure 5. The effective charge calculated by the modified empirical model is equal to 0.93. The value is consistent with the result presented in [Reference Zhang, Liu, Cheng, Zhao and He32], in which electrons are all assumed to be captured into the projectile ground state. Figure 5 represents the theoretical predictions from the modified empirical model, which are in agreement with the experimental data in the range of errors. It implied that the effective charge for 100 keV protons in plasmas also needs to be considered, as described in [Reference Zhang, Liu, Cheng, Zhao and He32]. It is not in accord with the case of neutral matter in [Reference Olson and Salop42]. Meanwhile, in our previous work, that the effective charge for 100 keV protons is chosen as 1 is arbitrary. However, the detailed and further experimental measurements are necessary, and how the target properties are added in the empirical formulae still needs further theoretical investigation.

Figure 5: Energy losses of 100 keV protons in hydrogen gas discharging plasma. Theoretical model calculations are also shown for comparison.
4. Summary
The energy losses of protons with the initial energy of 90 keV and 100 keV penetrating through the hydrogen plasma have been measured. The enhancement of energy loss in plasma compared to cold gas is introduced, which is consistent with our previous work. In our investigation, however, when Z eff = 1, the experimental data for 100 keV protons can be described by the theoretical predictions of the Bethe model, while it fails for 90 keV case, in which the theoretical calculations overestimate the experimental data. We apply the charge-state evolution to discuss our experimental results, and in the low-energy regime, the charge state remains 1 for larger than 100 keV protons, whereas the charge-state evolution needs to be considered with the decrease of incident energy. In order to reproduce the experimental results, the two empirical formulae are used to extract the effective charge. In the present work, the theoretical calculations from the effective charge extracted by two empirical formulae all underestimate the experimental data, which is mainly ascribed not to be referred to ionization degree of plasma in the empirical formulae. Based on our experimental results, a modification of the empirical formula is proposed, and the experimental data can be well reproduced. Moreover, the systematical measurement on energy loss and charge-state distribution for protons will be carried out in the future.
Data Availability
Data can be available upon request.
Conflicts of Interest
The authors declare that they have no conflicts of interest.
Acknowledgments
The work was supported by the Major State Basic Research Development Program of China (2017YFA0402300) and the National Natural Science Foundation of China (NSFC, Grant nos. 11775278, 11775042, 11875096, and U1532263). The authors sincerely acknowledge the technical supports by the HIFRL-ECR group.







