1. Introduction
The prospect of using organics as nonlinear optical materials for photonic switching and optical limiting applications has been the subject of many investigators during the past decade and has a great impact on information technology and industrial applications. In particular, the photonic applications such as all-optical switching, three-dimensional optical devices, all-optical limiting, medical science, and other optical fields [Reference Zyss1, Reference Munn and Ironside2]. Organic materials are most attractive owing to the known rich variety of organic compounds and the inherent flexibility in synthesizing such compounds with desired properties. The nonlinearity in these molecules has been found to originate from a strong delocalization of π-electrons along the length of the molecule [Reference Raghavendra, Chandra Shekhara Shetty and Chidan Kumar3, Reference Feng, Li and Shi4]. By molecular engineering, we can design organic systems for better nonlinear properties, and the study of the linear and nonlinear optical (NLO) coefficients helps us to identify such materials.
The third-order nonlinearity of conjugated organic compounds can be enhanced by (i) increasing the conjugation length, to increase the distance over which charge can be transferred; (ii) creating a donor-acceptor-donor motif by substitution, to increase the extent of charge transfer from the ends of the molecule to the center; and (iii) reversing the sense of symmetric charge transfer by substituting electron acceptors and donors, thereby creating acceptor-donor-acceptor compounds. The above strategy was used in the synthesis of the hydrazone derivatives, which are studied in this report, with a goal to enhance their nonlinear optical response by modifying the basic structure by substituting various electron donating and electron withdrawing groups [Reference Albota, Beljonne and Bredas5].
Hydrazone is an efficient class of organic materials for nonlinear optics. Since the hydrazone backbone is an asymmetric transmitter, it strongly increases the molecular nonlinearity for the electron donating and withdrawing group substitutions [Reference Pan, Wong, Bosch, Bosshard, Meier and Gunter6–Reference Xu, Shao, Han, Wang, Song and Hou8]. In this paper, we selected three hydrazone derivatives, namely, ethyl 2-((2E)-2-(4-(dimethylamino)benzylidene)hydrazinyl)-5-nitrobenzoate (H1), ethyl 2-((2E)-2-(4-chlorobenzylidene)hydrazino)-5-nitrobenzoate (H2), and methyl 5-nitro-2-((2E)-2-(4-nitrobenzylidene)hydrazino)benzoate (H3). Sample H1 is substituted with N-N-dimethyl amino group N(CH3)2, whereas samples H2 and H3 are substituted with Cl and NO2 groups, respectively. The N(CH3)2 and Cl groups are electron donors, whereas the NO2 group is an electron acceptor. Towards identifying efficient nonlinear optical materials, experimental methods such as Z-scan, pump probe, four wave mixing experiments are frequently used [Reference Anandan, Manoharan, Siji Narendranb and Sabari Girisunb9, Reference Habeeba, Saravanan, Girisun and Anandan10]. The single beam Z-scan technique is employed to investigate the third-order nonlinear optical properties in these materials. The compounds exhibit noticeable third-order nonlinearity and good optical limiting with nanosecond laser pulses. However, they cannot be directly used in practical devices because they are not flexible like polymers and are degraded when exposed to intense laser beams. To overcome this problem and make use of these materials in devices, the compounds were doped into the PMMA matrix. PMMA is a hard, rigid, and transparent nonlinear optical polymer with a glass transition temperature of 125°C. Its average molecular weight is 60,000. The physical durability of PMMA is far superior than that of other thermoplastics and is tougher than polystyrene. PMMA is most preferred for designing components because of its better resistance to hydrolysis, excellent environmental stability, easy handling and processing, and low cost [Reference Furniss, Hannaford, Smith and Tatchell11]. This can enhance the opto-chemical and opto-physical stability, as well as the mechanical and thermal properties, while retaining the NLO properties and linear optical transparency [Reference Billmeyer12].
In this article, the Z-scan technique is utilized to study the third-order NLO properties, and structure-property relationship of the hydrazone-based pure compounds in PMMA host using nanosecond laser pulses at 532 nm is reported. The optical limiting studies were also carried out. The relationship between molecular structure and the observed NLO behavior is investigated. In comparison with previously reported works on nonlinear optical coefficient of the PMMA/HQPQ-guest/host system and poly(((s)-1-(4-nitrophenyl)-2-pyrrolidinethyl)methacrylate) (PPM) in PMMA, the third-order nonlinear optical coefficient observed in the present work is one order higher in magnitude [Reference D’Amore, Lanata, Pietralunga, Gallazzi and Zerbi13, Reference Saadon14]. The experimental results reveal that these compounds could be tailored suitably for third-order NLO applications.
2. Experiment
The compounds were synthesized by using the standard procedure [Reference Furniss, Hannaford, Smith and Tatchell11]. Ethyl-2-hydrazino-5-nitrobenzoate (1.2 g, 0.005 mol) and appropriate aldehyde (0.005 mol) were dissolved in 25 ml of ethanol. To the cold solution, 3 drops of concentrated sulfuric acid was added. Then, the contents were refluxed on a water bath for 8 hrs. Excess ethanol was removed from the reaction mixture under reduced pressure. The solid product obtained was filtered, washed with water, dried, and recrystallized from ethanol—DMF mixture. The structure of the compounds is shown in Figure 1(a).

Figure 1: (a) Structure of the hydrazone compounds, (b) normalized absorption spectra of the studied compounds, and (c) schematic of the Z-scan experimental setup.
For doping, both the compound and PMMA were dissolved in dimethyl formamide (DMF) solution. The concentration of the dopant in the PMMA matrix was varied from 5 to 25%. Figure 1(b) shows the linear absorption spectra of the pure compounds recorded at room temperature in DMF solutions using a spectrophotometer (UV-2450 PC Series) and verified that the 532 nm laser excitation falls under nonresonant excitation regime. The linear refractive indices of the samples were measured using a Refracto 30GS digital refractometer. The measurements were performed with 1 × 10−3 mol/L concentration.
3. Z-Scan Measurements
The Z-scan technique is a simple but accurate method to determine both nonlinear index of refraction, n 2, and nonlinear absorption coefficient, β. Nonlinear index of refraction is proportional to the real part of third-order susceptibility Re [χ (3)], and the nonlinear absorption coefficient is proportional to the imaginary part, Im [χ (3)] [Reference Bredas, Adant, Tackx, Persoons and Pierce15]. The schematic of the Z-scan experimental setup is shown in Figure 1(c). By monitoring the transmittance through a small circular aperture placed at the far field position (closed aperture), one can determine the nonlinear refractive index. The nonlinear absorption coefficient of the sample can be determined using the open aperture (OA) Z-scan arrangement [Reference Sheik-Bahae, Said and VanStryland16, Reference Sheik-Bahae, Said, Wei, Hagan and VanStryland17].
A Q-switched Nd : YAG laser with a pulse width of 7 ns at 532 nm was employed in the experiment. A lens of focal length 23.5 cm was used to focus the laser pulses into a 1mm-quartz cuvette containing sample solution. The closed aperture (CA) Z-scan was performed with 50% aperture. In an attempt to suppress cumulative thermal effects, data were collected in the single shot mode [Reference Yang, Xu, Ballato, Schwartz and Carroll18]. Furthermore, to determine the effect of the solvent and PMMA to the observed NLO properties, we conducted the Z-scan experiment on the pure DMF and PMMA dissolved in DMF. It does not show any nonlinear effects.
4. Results and Discussion
The nonlinear transmission of the compounds with and without aperture was measured in the far field as the sample is moved through the focal point. The scan was performed at a laser pulse energy of 20 μJ, which corresponds to a peak intensity of 0.735 GW/cm2. Assuming a spatial and temporal Gaussian profile for laser pulses, the open aperture (OA) normalized energy transmittance is given by [Reference Sheik-Bahae, Said, Wei, Hagan and VanStryland17, Reference Sutherland19]
where β eff is the effective two photon absorption coefficient, I
0 is the input irradiance, z is the sample position,
![]() is the Rayleigh range, ω0
is the beam waist radius at the focal point(z = 0), and λ is the laser wavelength. For fitting the data with equation (1), we consider L eff as the effective path lengths in the case of 2PA and is defined by
is the Rayleigh range, ω0
is the beam waist radius at the focal point(z = 0), and λ is the laser wavelength. For fitting the data with equation (1), we consider L eff as the effective path lengths in the case of 2PA and is defined by
![]() , where L is the sample length and α 0 is the linear absorption coefficient. Similarly, for a pure nonlinear refraction curve, obtained by the division method, the normalized transmittance is given by [Reference Muruganandi, Saravanan, Vinitha, Jessie Raj and Sabari Girisun20–Reference Sabari Girisun, Saravanan and Soma22]
, where L is the sample length and α 0 is the linear absorption coefficient. Similarly, for a pure nonlinear refraction curve, obtained by the division method, the normalized transmittance is given by [Reference Muruganandi, Saravanan, Vinitha, Jessie Raj and Sabari Girisun20–Reference Sabari Girisun, Saravanan and Soma22]
where
![]() is the on-axis nonlinear phase shift at the focus and
is the on-axis nonlinear phase shift at the focus and
![]() . The nonlinear refractive index
. The nonlinear refractive index
![]() can be determined from the equation
can be determined from the equation
![]() , where k = 2π/λ is the wave vector and λ is the wavelength.
, where k = 2π/λ is the wave vector and λ is the wavelength.
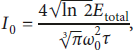
where E total is the incident energy on the sample after reflection from the front surface of the cuvette is taken into account and τ is the pulse width of the laser. The nonlinear refractive index n 2 (esu) can be calculated from the conversion formulae:

where n 0 the linear refractive index and c is the velocity of light in vacuum.
The normalized OA curve for the pure compounds and the compounds with PMMA (concentration of 25% in matrix) dissolved in DMF is shown in Figure 2. The solid lines are the theoretical fit of equation (1) to the experimental data, which yields the value of effective two photon absorption coefficient β eff.

Figure 2: Open aperture Z-scan curves for pure and PMMA-doped compounds. The open circles represent experimental data, while the solid line represents theoretical fit with β eff = 2 cm/GW, 0.0364 cm/GW, and 0.0258 cm/GW for H1, H2, and H3, respectively, and β eff = 2.2 cm/GW, 0.047 cm/GW, and 0.032 cm/GW for H1/PMMA, H2/PMMA, and H3/PMMA, respectively.
The pure nonlinear refraction curve obtained for pure compounds H1 and H1/PMMA is shown in Figure 3. Here, the solid lines are theoretical fit of the experimental data to equation (2).

Figure 3: Pure nonlinear curves obtained by the division method for pure compounds doped with PMMA. Solid lines are theoretical fit of the experimental data to equation (2).
The real and imaginary part of the third-order nonlinear optical susceptibility χ (3) can be calculated according to the following relations:
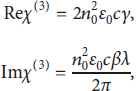
where n 0 is the linear refractive index, ε 0 is the vacuum permittivity, and c is the velocity of light in vacuum.
Second-order hyperpolarizability γ h which describes the nonlinear induced polarization per molecule, in an isotropic medium, is related to the third-order bulk susceptibility as [Reference Zhao, Singh and Prasad23–Reference Shivaraj, Parutagouda and Patila25]
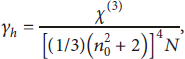
where N is the density of molecules in the unit of molecules per cm3, n 0 is the linear refractive index of the medium, and
![]() .
.
The values of γh for the pure compounds and the compounds in PMMA is of the order of 10−31 esu, which is well comparable with the value reported for silicon naphthalocyanine, SiNc
![]() , a widely known optical limiting material [Reference Guo, Sun, Wang, Zhao, Lu and Nie26]. The calculated values of n 2, Reχ (3), Im χ
(3), and γh for the pure compounds and compounds doped with PMMA are shown in Tables 1 and 2.
, a widely known optical limiting material [Reference Guo, Sun, Wang, Zhao, Lu and Nie26]. The calculated values of n 2, Reχ (3), Im χ
(3), and γh for the pure compounds and compounds doped with PMMA are shown in Tables 1 and 2.
Table 1: Values of Re χ (3), Im χ (3), n 2, and γ h determined experimentally for pure compounds.
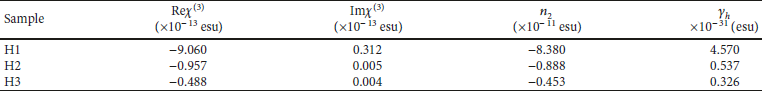
Table 2: Values of Re χ (3), Im χ (3), n 2, and γ h determined experimentally for the compounds doped with PMMA.

The dependence of the nonlinear absorption (NLA) coefficient with on-axis irradiance gives the information about the mechanism of the nonlinear absorption. Generally, nonlinear absorption can be caused by free carrier absorption, saturable absorption, and direct multiphoton absorption or excited state absorption. From our observations, it is seen that β eff is decreasing with increasing intensity for all the compounds and intercept on the vertical axis is nonzero, as shown in Figure 4. The fall of β eff with increasing I 0 is a consequence of reverse saturable absorption (RSA) [Reference Guo, Xu, Wang, You and Ming27, Reference Couris, Koudoumas, Ruth and Leach28]. RSA generally arises in a molecular system where the presence of excited-state absorption to a higher lying state occurs. In particular, when the excited state absorption cross section is larger than the ground state cross section [Reference Torres-Torres, Khomenko, Tamayo-Rivera, Rangel-Rojo, Mao and Watson29]. To confirm the presence of RSA, we calculated the absorption cross-sections in the ground and excited states. The excited state cross section σ ex can be calculated from the normalized Z-scan data as the procedure described in the literature [Reference Henari, Blau, Milgrom, Yahioglu, Phillips and Lacey30, Reference Garoni, Colombo, Kamada, Dragonetti and Roberto31].
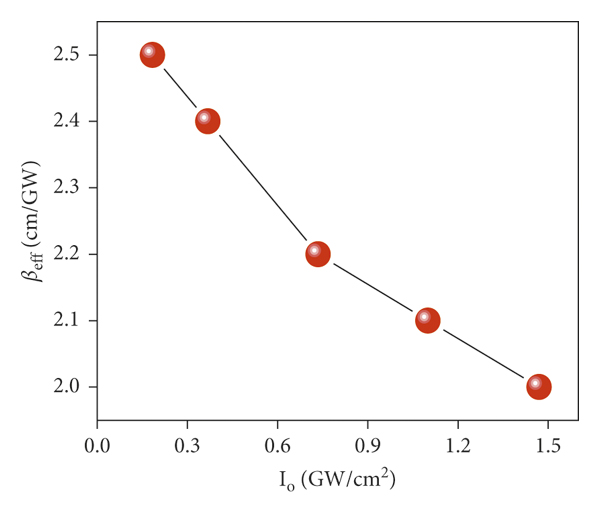
Figure 4: Variation of β eff with on-axis intensity I 0 within the compound H1.
The ground state absorption cross section σg can be calculated from the following equation:
where Na is the Avogadro number, C is the concentration in moles/cm3, and α is the linear absorption coefficient.
The calculated values of σ ex for the pure compounds H1, H2, and H3 are 1.522 × 10−17 cm2, 1.475 × 10−18 cm2, and 1.43 × 10−18 cm2, respectively. For compounds H1, H2, and H3 doped with PMMA, the values of σ ex are 1.739 × 10−17 cm2, 1.656 × 10−18 cm2, and 1.50 × 10−18 cm2, respectively. The values of σg for the compounds H1, H2, and H3 are calculated as 2.075 × 10−18 cm2, 8.30 × 10−21 cm2, and 1.66 × 10−21 cm2, respectively. It is seen that the value of σex is larger than the value of σg for both pure compounds and compounds doped with PMMA which is in agreement with the condition for reverse saturation absorption (RSA) [Reference Tutt and Boggess32].
The dependence of β eff on the concentration of the sample in the polymer matrix is shown in Figure 5.

Figure 5: βeff vs. concentration of the sample H1 in the polymer matrix.
Based on the strong reverse saturated absorption, a good optical limiting of nanosecond laser pulses of the samples can be expected. Figure 6 shows the optical limiting behaviour of the compound doped with PMMA. It can be seen that the best limiting behaviour is observed with sample H1, which exhibits strongest nonlinear absorption among the samples. For incident energies less than 100 μJ/pulse, the output linearly increases with the input. But for energies more than 100 μJ/pulse, optical limiting of the pulses was observed. For input energies well below 250 μJ, there is no damage observed in the samples. But beyond 300 μJ, there is a deviation from the optical limiting behaviour. It could be due to damage of sample with laser pulses.
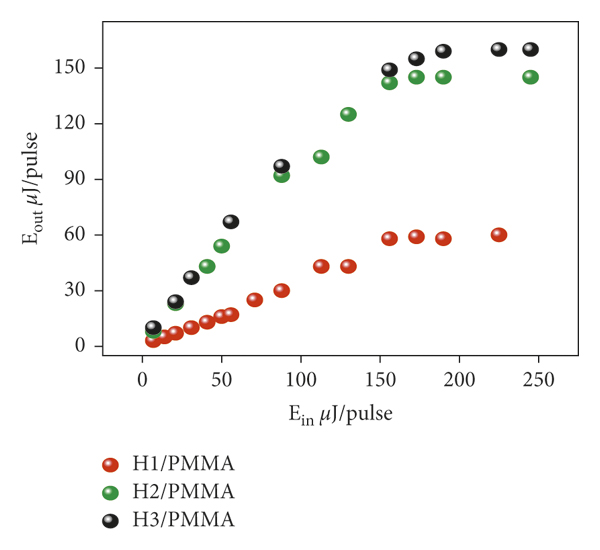
Figure 6: Optical limiting behavior in the compounds doped with PMMA.
These results show that the compounds exhibit larger third-order NLO properties in PMMA host as compared to the pure compounds. The π-electrons associated with the dopant molecules will form an electron cloud around the chain and can be distorted by applying an electric field, which results in variation of nonlinear effects in the samples. Even though the enhancement of NLO properties is very low, PMMA is most preferred because of its flexibility and and good resistance having a low density that aids fabrication of lightweight components, and it increases the damage threshold of the compounds.
From the results, we noticed that the nonlinear response among the three hydrazone derivatives is of the order H1 > H2 > H3. In the case of H1, the highly electron donating dimethyl amino group—N(CH3)2—is attached to the para position. Thus, compared to the acceptor group on the left-hand side, in which the carbon ring is attached to the highly electron accepting NO2 at the metaposition and ester group at the ipso position, the right-hand side of the molecule acts as a donor giving away electrons easily to form a stable π-electron distributed system. Hence, there is a strong delocalization of electrons in the molecule that gives rise to the large nonlinear polarization. Consequently, the highest nonlinear response was observed with H1. In case of H2, chlorine atom is attached at the para position. Cl is an amphiprotic group, and its contribution to the molecular hyperpolarizability depends on the strength of the acceptor group attached to the phenyl ring of the molecule [Reference Honark33]. Since the acceptor group at the left-hand side is very strong, the Cl atoms acts as a donor. But in H3, a strong acceptor NO2 group is attached at the para position. The NO2 groups at both ends accept electrons, and hence, delocalization decreases in the molecule.
It can be noted that enhancement of PMMA nonlinear optical properties by means of a quinoid molecule was reported with χ (3) values of 10−14 esu [Reference D’Amore, Lanata, Pietralunga, Gallazzi and Zerbi13]. It is also noted that χ (3) value of ethyl 2-((2E)-2-(4-(dimethylamino)benzylidene)hydrazinyl)-5-nitrobenzoate is comparable with the values of the order of 10−13 esu of many varieties of azo dyes, which are well-known third-order nonlinear optical materials [Reference Ghanavatkar, Mishra and Sekar34]. Thus, the work reported here reveals that these compounds are one of the suitable classes of nonlinear optical material for photonic applications.
5. Conclusions
The third-order nonlinear properties of hydrazone derivatives with different substituents were investigated using the single-beam Z-scan technique with 7 ns laser pulses at 532 nm. For making effective use of this materials in devices, the compounds were doped into poly(methylmethacrylate), and the third-order optical properties were studied. The results show that the compounds exhibit larger third-order NLO properties in PMMA host as compared to the pure compounds. The calculated values of nonlinear refractive index and second-order hyperpolarizability are of the order of 10−11 esu and 10−31 esu, respectively. Hence, these hydrazones can be suitable materials for photonic applications.
Data Availability
Data underlying the results presented in this paper are not publicly available at this time but may be obtained from the corresponding author upon reasonable request.
Conflicts of Interest
The authors declare no conflicts of interest.
Acknowledgments
S. K acknowledges Kuwait Foundation for the Advancement of Sciences (KFAS), Kuwait, for the research grant (no:PR18-13SP-01). E. Shiju acknowledges SERB, India, for providing financial assistance through NPDF (PDF/2020/002494). This research was funded by the Kuwait Foundation for the Advancement of Sciences (KFAS), Kuwait (grant no.PR18-13SP-01).









