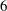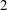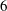Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Shatsila, Anatoli
2023.
Geometry of elliptic normal curves of degree 6.
Communications in Algebra,
Vol. 51,
Issue. 12,
p.
5344.