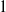Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Li, Xiaoyue
Mao, Xuerong
and
Song, Guoting
2024.
An explicit approximation for super-linear stochastic functional differential equations.
Stochastic Processes and their Applications,
Vol. 169,
Issue. ,
p.
104275.