Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-13T10:46:28.819Z Has data issue: false hasContentIssue false
  • English
  • Français

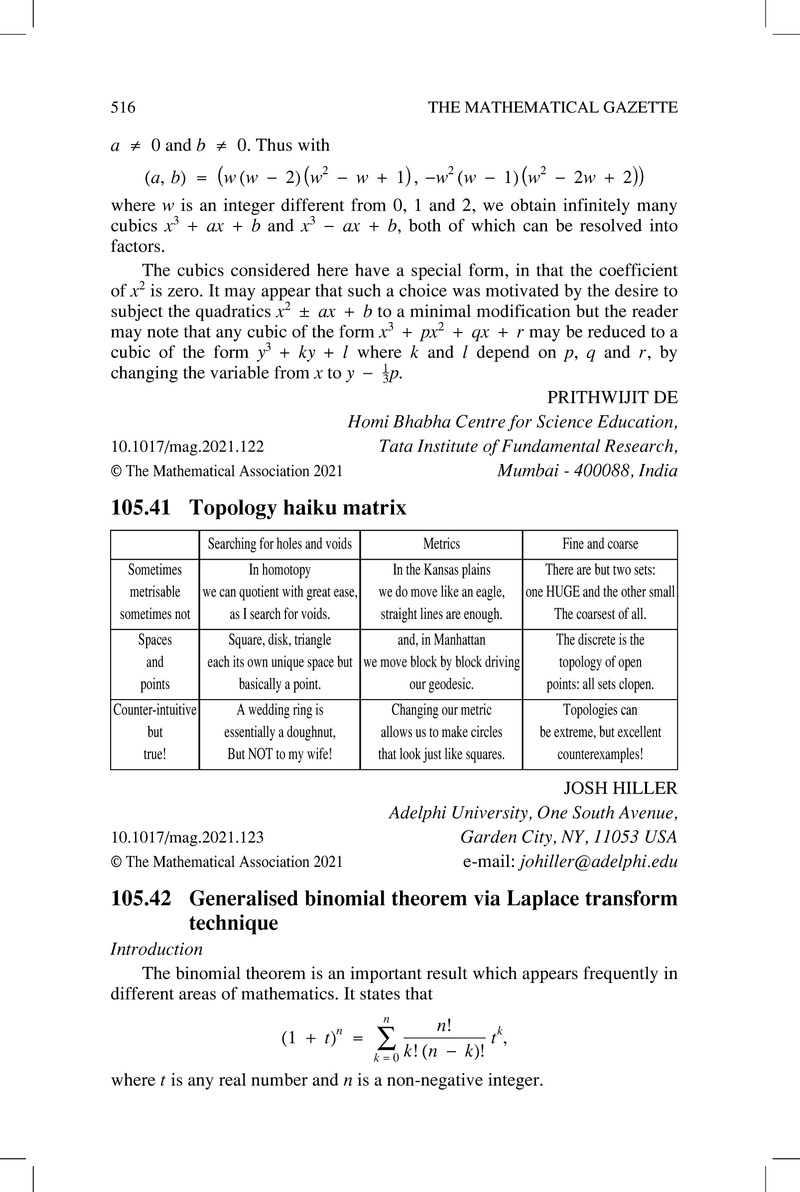
105.42 Generalised binomial theorem via Laplace transform technique

Published online by Cambridge University Press:  13 October 2021

Kuldeep Kumar Kataria  and
Raj Kumar Mistri
Kuldeep Kumar Kataria
Affiliation:
Department of Mathematics, Indian Institute of Technology Bhilai, Raipur-492015, India, e-mails: kuldeepk@iitbhilai.ac.in
Raj Kumar Mistri
Affiliation:
Department of Mathematics, Indian Institute of Technology Bhilai, Raipur-492015, India, e-mails: rkmistri@iitbhilai.ac.in
Get access Rights & Permissions [Opens in a new window]

Abstract

An abstract is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Notes
Information
The Mathematical Gazette , Volume 105 , Issue 564 , November 2021 , pp. 516 - 520
Check for updates
Copyright
© The Mathematical Association 2021

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Coolidge, J. L., The story of the binomial theorem, Amer. Math. Monthly 56 (1949) pp. 147-157.CrossRefGoogle Scholar
Smoryński, C., A treatise on the binomial theorem, Texts in mathematics, College Publications, London (2012).Google Scholar
Goss, D., The ongoing binomial revolution, In: F. Bars, Longhi, I., Trihan, F., (eds) Arithmetic geometry over global function fields, Advanced Courses in Mathematics, CRM Barcelona, Birkhauser, Basel, (2014).Google Scholar
Fulton, C. M., A simple proof of the binomial theorem, Amer. Math. Monthly 59 (1952) pp. 243-244.CrossRefGoogle Scholar
Rosalsky, A., A simple and probabilistic proof of the binomial theorem, Amer. Statist. 61 (2007) pp. 161-162.CrossRefGoogle Scholar
Hwang, L. C., A simple proof of the binomial theorem using differential calculus, Amer. Statist. 63 (2009) pp. 43-44.Google Scholar
Kataria, K. K., The binomial theorem procured from the solution of an ODE, Math. Magazine 90 (2017) pp. 375-377.10.4169/math.mag.90.5.375CrossRefGoogle Scholar
Kataria, K. K., An alternate proof of the binomial theorem, Amer. Math. Monthly 123 (2016) p. 940.CrossRefGoogle Scholar
Rudin, W., Principles of mathematical analysis (3rd edn.), McGraw-Hill, New York (1976).Google Scholar