Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Al Rabee, Faeik T.
Al-Rimawi, Ashraf
and
Gitlin, Richard D.
2018.
Channel Capacity in a Dynamic Random Waypoint Mobility Model.
p.
711.
Mayer, Alexander
2019.
Estimation and Inference in Adaptive Learning Models with Slowly Decreasing Gains.
SSRN Electronic Journal,
Eggers, Patrick C. F.
Angjelichinoski, Marko
and
Popovski, Petar
2019.
Wireless Channel Modeling Perspectives for Ultra-Reliable Communications.
IEEE Transactions on Wireless Communications,
Vol. 18,
Issue. 4,
p.
2229.
Abolhassani, Bahman
Tadrous, John
and
Eryilmaz, Atilla
2019.
Wireless Multicasting for Content Distribution: Stability and Delay Gain Analysis.
p.
1.
Smetana, Kathrin
Zahm, Olivier
and
Patera, Anthony T.
2019.
Randomized Residual-Based Error Estimators for Parametrized Equations.
SIAM Journal on Scientific Computing,
Vol. 41,
Issue. 2,
p.
A900.
Atta, A. G.
Moatimid, G. M.
and
Youssri, Y. H.
2019.
Generalized Fibonacci Operational Collocation Approach for Fractional Initial Value Problems.
International Journal of Applied and Computational Mathematics,
Vol. 5,
Issue. 1,
Akkouchi, Mohamed
and
Ighachane, Mohamed Amine
2019.
Refinements of Some Recent Inequalities for Certain Special Functions.
Annales Mathematicae Silesianae,
Vol. 33,
Issue. 1,
p.
1.
Bai, Tong
Wang, Jingjing
Ren, Yong
and
Hanzo, Lajos
2019.
Energy-Efficient Computation Offloading for Secure UAV-Edge-Computing Systems.
IEEE Transactions on Vehicular Technology,
Vol. 68,
Issue. 6,
p.
6074.
Mahata, Shantiram
and
Sinha, Rajen Kumar
2020.
On the existence, uniqueness and stability results for time-fractional parabolic integrodifferential equations.
Journal of Integral Equations and Applications,
Vol. 32,
Issue. 4,
Chavez, Gordon V.
2020.
Dynamic tail inference with log-Laplace volatility.
Extremes,
Vol. 23,
Issue. 2,
p.
287.
Beghin, Luisa
and
Gajda, Janusz
2020.
Tempered Relaxation Equation and Related Generalized Stable Processes.
Fractional Calculus and Applied Analysis,
Vol. 23,
Issue. 5,
p.
1248.
Liu, Siqi
Melia, Adrian
Song, Xiaojing
and
Tippett, Mark
2020.
Singular diffusions, constant elasticity of variance processes and logarithmic rates of return.
The European Journal of Finance,
Vol. 26,
Issue. 9,
p.
837.
Tarable, Alberto
and
Escribano, Francisco J.
2020.
Chaos-Based Anytime-Reliable Coded Communications.
IEEE Systems Journal,
Vol. 14,
Issue. 2,
p.
2214.
Kakavas-Papaniaros, P. A.
2020.
On the distribution of particles in propellant solids.
Acta Mechanica,
Vol. 231,
Issue. 3,
p.
863.
Mathias, Simon A.
Dentz, Marco
and
Liu, Qingquan
2020.
Gas Diffusion in Coal Powders is a Multi-rate Process.
Transport in Porous Media,
Vol. 131,
Issue. 3,
p.
1037.
Morales, Guillermo B.
and
Muñoz, Miguel A.
2021.
Immune amnesia induced by measles and its effects on concurrent epidemics.
Journal of The Royal Society Interface,
Vol. 18,
Issue. 179,
p.
20210153.
Miao, Maoke
and
Li, Xiaofeng
2021.
Novel approximate distribution of the sum of Gamma–Gamma variates with pointing errors and applications in MIMO FSO links.
Optics Communications,
Vol. 486,
Issue. ,
p.
126780.
Das, Pratibhamoy
and
Rana, Subrata
2021.
Theoretical prospects of fractional order weakly singular Volterra Integro differential equations and their approximations with convergence analysis.
Mathematical Methods in the Applied Sciences,
Vol. 44,
Issue. 11,
p.
9419.
Iranmanesh, Saeid
Abkenar, Forough Shirin
Raad, Raad
and
Jamalipour, Abbas
2021.
Improving Throughput of 5G Cellular Networks via 3D Placement Optimization of Logistics Drones.
IEEE Transactions on Vehicular Technology,
Vol. 70,
Issue. 2,
p.
1448.
Kremer, D.
and
Scheffler, H.-P.
2021.
Multivariate tempered stable random fields.
Journal of Mathematical Analysis and Applications,
Vol. 503,
Issue. 2,
p.
125347.
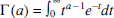 for a > 0. By splitting this integral at a point x ⩾ 0, we obtain the two incomplete gamma functions:
for a > 0. By splitting this integral at a point x ⩾ 0, we obtain the two incomplete gamma functions: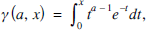 (1)
(1)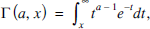 (2)
(2)
