No CrossRef data available.
Published online by Cambridge University Press: 14 June 2016
The behaviour of the divisor function d (n) is rather tricky. For a prime p, we have d(p) = 2, but if n is the product of the first k primes then, by Chebyshev's estimate for the prime counting function [1, Theorem 414], we have 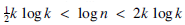 so that
so that

for such n then, d (n) is ‘unusually large’ — it can exceed any fixed power of log n, for example.
In [2] Jameson gives, amongst other things, a derivation of Dirichlet's theorem, which shows that the mean-value of the divisor function in an interval containing n is log n. However, the result is somewhat deceptive because, for most n, the value of d (n) is substantially smaller than log n.