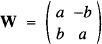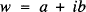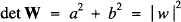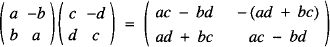Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Lantoine, Gregory
Russell, Ryan P.
and
Dargent, Thierry
2012.
Using Multicomplex Variables for Automatic Computation of High-Order Derivatives.
ACM Transactions on Mathematical Software,
Vol. 38,
Issue. 3,
p.
1.