Article contents
Approximating Lipschitz and continuous functions by polynomials; Jackson’s theorem
Published online by Cambridge University Press: 03 July 2023
Extract
The celebrated theorem of Weierstrass, dating from 1885, states that continuous functions can be uniformly approximated by polynomials on any bounded, closed interval. But just how well can we approximate by polynomials of a certain degree? Let us introduce some notation to facilitate the discussion. For an interval I (which will usually be 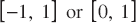 ), denote by C (I) the space of continuous functions on I, and write
), denote by C (I) the space of continuous functions on I, and write 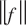 for
for  (the notation
(the notation 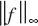 is often used). Uniform convergence of fn to f (on I) equates to the statement that
is often used). Uniform convergence of fn to f (on I) equates to the statement that 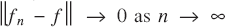 . Denote by
. Denote by 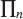 the space of polynomials of degree not more than n. This is a linear subspace of C (I) of dimension n + 1. We write
the space of polynomials of degree not more than n. This is a linear subspace of C (I) of dimension n + 1. We write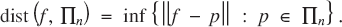
- Type
- Articles
- Information
- Copyright
- © The Authors, 2023. Published by Cambridge University Press on behalf of The Mathematical Association
References
- 1
- Cited by



