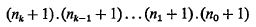Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-28T06:09:51.176Z Has data issue: false hasContentIssue false
  • English
  • Français

Divisibility properties of binomial coefficients

Published online by Cambridge University Press:  03 November 2016

K. R. McLean
K. R. McLean*
Affiliation:
School of Education, Liverpool UniversityL69 3BX
Get access Rights & Permissions [Opens in a new window]

Extract

In a very interesting recent article [1] W. A. Broomhead described an investigation carried out by staff and pupils at Tonbridge School of the patterns which result when the numbers in Pascal’s triangle are reduced modulo m. For the case when m equals a prime number, p, the pattern formed by the zeros in the reduced triangle (corresponding to binomial coefficients divisible p) was completely described and the following result (stated by G. Gilbart-Smith) was proved:

In the (n + l)th row of Pascal’s triangle, there are

Type
Research Article
Information
The Mathematical Gazette , Volume 58 , Issue 403 , March 1974 , pp. 17 - 24
Copyright
Copyright © Mathematical Association 1974

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Broomhead, W. A., Pascal (mod p), Mathl Gaz. LVI, 267–271 (No. 398, December 1972).Google Scholar
2. Lockwood, E. H. and Gant, P., Factors of binomial coefficients, Mathl Gaz. XLIII, 31–34 (No. 343, February 1959).Google Scholar