Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-13T10:41:38.154Z Has data issue: false hasContentIssue false
  • English
  • Français

Farey rabbits

Published online by Cambridge University Press:  01 August 2016

Abraham Arcavi  and
Maxim Bruckheimer
Abraham Arcavi
Affiliation:
Department of Science Teaching, Weizmann Institute of Science, Rehovot 76100, Israel
Maxim Bruckheimer
Affiliation:
Department of Science Teaching, Weizmann Institute of Science, Rehovot 76100, Israel
Get access Rights & Permissions [Opens in a new window]

Extract

The Farey sequence of order n(Fn) is the sequence of all reduced fractions between 0 and 1, whose denominator does not exceed n, arranged in increasing order of magnitude.

For example, F6 is .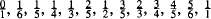

The Fibonacci sequence is the sequence for which u1 = 1, u2 = 1 and un = un-1 + un-2,namely, 1, 1, 2, 3, 5, 8, 13, … , un

At first sight, there is little connection between Farey’s fractions and Fibonacci’s integers. The purpose of this note is to show and explore such a connection and hence derive some properties of the Fibonacci sequence directly from previously proved properties of the Farey sequences. The former properties are well-known, but their rather unusual derivation from Farey properties may have some interest.

Type
Research Article
Information
The Mathematical Gazette , Volume 84 , Issue 500 , July 2000 , pp. 223 - 226
Copyright
Copyright © The Mathematical Association 2000

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Bruckheimer, M. and Arcavi, A., A visual approach to some elementary number theory, Math. Gaz. 79 (November 1995) pp. 471–478.CrossRefGoogle Scholar
2. Borob’ev, N. N., Fibonacci numbers. Pergamon Press 1961.Google Scholar