No one can possibly welcome more cordially than I do the widening of the school curriculum in mathematics that has taken place during the last twenty years. Even I can remember the days when ‘Conics’ and ‘Mechanics’ were the privilege of a select few, and only a prodigy was initiated into the mysteries of the Differential (much less the Integral) Calculus. All this is changed now, and it is fairly safe (at least that is my experience) to assume that a boy who has won a scholarship has learnt something about the Integral Calculus at school.
page 99 of note * Berry, A., Messenger of Mathematics, vol. 37, p. 61 Google Scholar; E. J. Nanson, ibid. p. 113.
page 99 of note † Whether this is true of a proof by ‘contour integration’ depends logically on what proof of Cauchy’s Theorem has been used (see my remarks under 3 below).
page 99 of note ‡ For an explanation of the general nature of a ‘double-limit problem’ see my Course of Pure Mathematics (App. 2, p. 420); for examples of the working out of such problems see Mr. Bromwich’s Infinite Series (passim, but especially App. 3, p. 414 et seq.).
page 100 of note * I mean, of course, the first proof mentioned by Mr. Berry—the proof itself is classical.
page 100 of note † Forsyth, , Theory of Functions, p. 23.Google Scholar
page 101 of note * Goursat’s proof is better and more general, and does not involve my inversion of limit-operations, but its difficulties are far too delicate for beginners.
page 102 of note * This proof is also referred to by Prof. Nanson. The only proofs which are ‘classical’ are 1, 2, 3, viz. the three discussed by Mr. Berry.
page 103 of note * This I know to be Mr. Berry’s opinion. He would put 2 below 3 and 4, and 5 below 6. To compare 3 and 4 is very difficult, and they might fairly be bracketed.
Since writing this note I have recollected another proof which I had forgotten, and which is given in § 173 (Ex. 1) of Mr. Bromwich’s Infinite Series. Mr. Bromwich there proves that, provided the real parts of a and b are positive or zero,
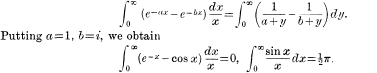
This proof, as presented by Mr. Bromwich, should be marked at about 45. It has the advantage of giving the values of several other interesting integrals as well, so that (as in the case of Prof. Nanson’s proof) it is hardly fair to contrast this mark with the considerably lower marks obtained by some of the other proofs.