No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Published online by Cambridge University Press: 03 November 2016
In this article an attempt has been made to indicate the ordinary methods of integration of the total differential equation
Then the condition for integrability of (1) , i.e, the condition that (1) may possess a single integral equivalent of the form ϕ(x, y, z)= c, is
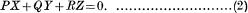
page 105 of note * References are to pages in the undermentioned works, and are abbreviated as follows : B. : Boole, G. Differential Equatzons, 4th ed. (1877).Google Scholar FI : Forsgth, A.R., Treatise on Differential Eqzirttzons, 6th ed. (1929).Google Scholar I .: Forsvth, A.R.. Theory of Differential Enuatlons. Part I (1890).Google Scholar G. : Goursag Cows, E. l. 11, 4th ed. (1925).Google Scholar I. : Ince, E.L., Ordinary Dzfferentinl Equations (1927).Google Scholar P : Piaggio, H.T.H., Treatise on Differential Equations, revised ed. (1825).Google Scholar W : Wilson, E.B., Advanced Calculus (1911).Google Scholar
page 108 of note * See also the footnote to section VI.
page 109 of note * Though not usually desirable as a practical method of integration, after λ has been found, instead of proceeding to the final integration, this method may be used in conjunction with method IV Thus, if µ and µ, have the values given in IV (ii). the above integral may be written in either of the forms
