Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Little, Charles H. C.
Teo, Kee L.
and
van Brunt, Bruce
2022.
An Introduction to Infinite Products.
p.
229.
Kalman, Dan
and
Currie, Mel
2025.
Geometric Extensions of a Mysterious Pattern.
The College Mathematics Journal,
Vol. 56,
Issue. 3,
p.
180.

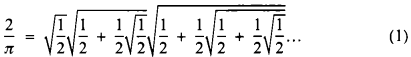

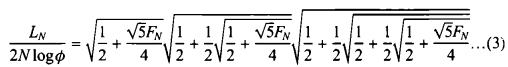
 is the golden section. (The Fibonacci numbers are
is the golden section. (The Fibonacci numbers are  , while the Lucas numbers are
, while the Lucas numbers are 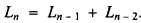 )
)