Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-11T04:43:35.448Z Has data issue: false hasContentIssue false
  • English
  • Français

xy = cos (x + y) and other implicit equations that are surprisingly easy to plot

Published online by Cambridge University Press:  15 February 2024

Michael Jewess
Michael Jewess*
Affiliation:
The Long Barn, Townsend, Harwell, Oxfordshire OX11 0DX e-mail: michaeljewess@researchinip.com
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The following equations relate y only implicitly to x:(1)(2)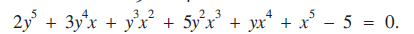 In both equations, y is a function of x for a continuous range of (x, y) values in the real x-y plane. (1) represents an ellipse. (2) has been designed by the author to have a solution in the real x-y plane at (−1, 2), and because the function on the left-hand side of (2) meets certain conditions regarding continuity and partial differentiability there must be a line of points in the real x-y plane satisfying (2) and passing continuously through (−1, 2) [1, pp. 23-28].

Type
Articles
Information
The Mathematical Gazette , Volume 108 , Issue 571 , March 2024 , pp. 1 - 11
Check for updates
Copyright
© The Authors, 2024 Published by Cambridge University Press on behalf of The Mathematical Association

References

Hilton, P. J., Partial derivatives, Routledge & Kegan Paul (1960) pp. 23–28.CrossRefGoogle Scholar
Kline, Morris, Mathematical thought from ancient to modern times Vol. 2 Oxford University Press, pbk. (1990) pp. 605-606, pp. 754-763. This summarises work on equations of degree ≥ 5 from 1799 to 1879.Google Scholar
Tall, David, Drawing implicit functions, Mathematics in School 15(2) (1986) pp. 33-37. This suggested how one might write one’s own programs on an early affordable desktop computer, and explains the algorithm used.Google Scholar
Mathworks, fimplicit, accessed October 2023 at https://uk.mathworks.com/help/matlab/ref/fimplicit.html This explains how, with no understanding of the algorithm, one may use ‘fimplicit’ in proprietary software to plot an implicit equation, merely by typing in the equation, slightly encoded.Google Scholar
Godfrey, C. and Siddons, A. W., Four-figure tables (new edn., corrected reprint), Cambridge University Press (1962). Godfrey and Siddons was a slim text dating back to 1913 which any student could afford, with at least one comparably venerable competitor. It included tables of logarithms (base 10), anti-logarithms (base 10), the six trigonometrical functions, logarithms (base 10) of those six functions, squares, square roots, reciprocals, logarithms (base e), e x, e −x, sinh x and cosh x. No tables of powers other than of 2 and were included, but users would have raised numbers to other powers by use of the logarithm and antilogarithm tables. For instance, 20.5613 would have been calculated as antilog10 (13 × log10 20.56), and . The complex roots are not obtained, but for the purposes of plotting in the real x-y plane, this is perfectly satisfactory.Google Scholar
Wikipedia, Mechanical calculator, accessed October 2023 at https://en.wikipedia.org/wiki/Mechanical_calculator. Some machines produced a paper record of what values and operations had been typed in together with the results obtained; this enormously facilitated checking.Google Scholar
John, W. Harris and Horst Stocker, Handbook of mathematics and computational science, Springer (1998) p. 321. Google Scholar