Published online by Cambridge University Press: 24 October 2008
A few years ago, the authors considered briefly Banach algebras whose duals could be identified ‘naturally’ with their multiplier algebras [17]. In this context, naturalness can be interpreted as meaning that, for each element b of the algebra B and each pair of elements u, v of the dual B′,
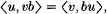
where 〈, 〉 denotes the dual pairing and the products are of elements of B′ regarded as left or right multipliers on B. In the present paper we return to the same circle of ideas but begin with a more general situation. We assume only that the algebra B is injectively embedded in its algebra of left, and in its algebra of right, multipliers and that its dual B′ can be injectively embedded in the algebra M(B) of double multipliers on B (definition below) in such a way that the above relation holds. From these assumptions we shall prove that there is a normed algebra A such that M(B) is the dual of A and is the algebra of continuous left multipliers on A (or, equally, right multipliers).