Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Conway, J. H.
and
Sloane, N. J. A.
1983.
Complex and integral laminated lattices.
Transactions of the American Mathematical Society,
Vol. 280,
Issue. 2,
p.
463.
Conway, J. H.
and
Sloane, N. J. A.
1984.
On the Voronoi Regions of Certain Lattices.
SIAM Journal on Algebraic Discrete Methods,
Vol. 5,
Issue. 3,
p.
294.
1987.
Geometry of Numbers.
Vol. 37,
Issue. ,
p.
632.
Kostrikin, A. I.
and
Chubarov, I. A.
1988.
Representations of finite groups.
Journal of Soviet Mathematics,
Vol. 40,
Issue. 3,
p.
331.
Kondrat'ev, A. S.
Makhnev, A. A.
and
Starostin, A. I.
1989.
Finite groups.
Journal of Soviet Mathematics,
Vol. 44,
Issue. 3,
p.
237.
Ignatenko, V. F.
1991.
Geometric theory of invariants of groups generated by reflections.
Journal of Soviet Mathematics,
Vol. 55,
Issue. 6,
p.
2168.
Rudnitskii, O. I.
1993.
Basis invariants of the Mitchell group generated by reflections in six-dimensional unitary space.
Journal of Soviet Mathematics,
Vol. 65,
Issue. 1,
p.
1479.
Bachoc, Christine
1995.
Voisinage au sens de Kneser pour les réseaux quaternioniens.
Commentarii Mathematici Helvetici,
Vol. 70,
Issue. 1,
p.
350.
Calderbank, A.R.
and
Sloane, N.J.A.
1996.
The ternary Golay code, the integers mod 9, and the Coxeter-Todd lattice.
IEEE Transactions on Information Theory,
Vol. 42,
Issue. 2,
p.
636.
Bachoc, Christine
1997.
Applications of Coding Theory to the Construction of Modular Lattices.
Journal of Combinatorial Theory, Series A,
Vol. 78,
Issue. 1,
p.
92.
Allcock, Daniel
2000.
New complex- and quaternion-hyperbolic reflection groups.
Duke Mathematical Journal,
Vol. 103,
Issue. 2,
Camastra, F.
and
Vinciarelli, A.
2002.
Estimating the intrinsic dimension of data with a fractal-based method.
IEEE Transactions on Pattern Analysis and Machine Intelligence,
Vol. 24,
Issue. 10,
p.
1404.
Hiltunen, J.
Hollanti, C.
and
Lahtonen, J.
2005.
Dense full-diversity matrix lattices for four transmit antenna MISO channel.
p.
1290.
Shukla, P.
and
Dragotti, P.L.
2005.
Sampling schemes for 2-D signals with finite rate of innovation using kernels that reproduce polynomials.
p.
II.
S. Tee, R.
Alamri, O.
and
Hanzo, L.
2006.
Joint Design of Twin-Antenna Assisted Space-Time Multilevel Sphere Packing Aided Coded Modulation.
p.
1.
Ben-Haim, Yael
and
Litsyn, Simon
2006.
Improved Upper Bounds on the Reliability Function of the Gaussian Channel.
p.
709.
Tee, R. Y. S.
Alamri, O.
Ng, S. X.
and
Hanzo, L.
2007.
Equivalent-Capacity-Based Design of Space-Time Block-Coded Sphere-Packing-Aided Multilevel Coding.
p.
4173.
Garbagnati, Alice
and
Sarti, Alessandra
2007.
Symplectic automorphisms of prime order on K3 surfaces.
Journal of Algebra,
Vol. 318,
Issue. 1,
p.
323.
Artebani, Michela
and
Sarti, Alessandra
2008.
Non-symplectic automorphisms of order 3 on K3 surfaces.
Mathematische Annalen,
Vol. 342,
Issue. 4,
p.
903.
Davis, Donald M.
2008.
Homotopy type and v1-periodic homotopy groups of p-compact groups.
Topology and its Applications,
Vol. 156,
Issue. 2,
p.
300.
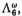 its automorphism group (which is Mitchell's reflection group 6·PSU(4, 3)·2), and the associated 12-dimensional real lattice K12. We give several constructions for
its automorphism group (which is Mitchell's reflection group 6·PSU(4, 3)·2), and the associated 12-dimensional real lattice K12. We give several constructions for 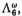 , which is a Z[ω]-lattice where ω = e2πi/3; enumerate the congruence classes of
, which is a Z[ω]-lattice where ω = e2πi/3; enumerate the congruence classes of  and
and  where θ = ω − ω¯; prove the lattice is unique; determine its covering radius and deep holes; and study its connections with the lattice E6 and the Leech lattice. A number of new dense lattices in dimensions up to about 107 are constructed. We also give an explicit basis for the invariants of the Mitchell group. The paper concludes with an extensive bibliography.
where θ = ω − ω¯; prove the lattice is unique; determine its covering radius and deep holes; and study its connections with the lattice E6 and the Leech lattice. A number of new dense lattices in dimensions up to about 107 are constructed. We also give an explicit basis for the invariants of the Mitchell group. The paper concludes with an extensive bibliography.

