Published online by Cambridge University Press: 24 October 2008
It is well known that the boundary value problem for the non-linear differential equation

can be reduced with help of a Green's function K (x, ξ) to a non-linear integral equation of the type
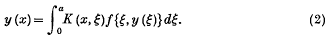
* Picard, E., Journ. de Math. (4), 9 (1893), 217.Google Scholar
† Hammerstein, A., Acta Math. 54 (1930), 118–22.CrossRefGoogle Scholar
† A similar argument in another connection was used by Signorini, Palermo Rend. 33 (1912), 187–93; Birkhoff, , Trans. Amer. Math. Soc. 18 (1912), 199–300CrossRefGoogle Scholar; and especially Morse, M., Trans. Amer. Math. Soc. 27 (1925), 213–34.Google Scholar
‡ See (a) Bogoliouboff, N. and Kryloff, N., Annals of Math. (2), 29 (1928), 255–75CrossRefGoogle Scholar, and (b) Kryloff, N., Tôhoku Math. Journ. 28 (1927), 65–8.Google Scholar In the latter paper our problem is treated under the condition 0 ≤ f ν (x, y) ≤ A with the help of Ritz' method; the error estimation is reduced to that of some other trigonometrical approximation of the solution.
§ We can replace this hypothesis by assuming only that f ν (x, y) is bounded, if, instead of differentiating with regard to a, in (iv), we use a geometrical argument due to R. Brauer (see Bieberbach, , Differentialgleichungen, Berlin, 3 Aufl. p. 197).Google Scholar
* Compare E. Picard, loc. cit.
† These functions were called y r (x; a r, a r−1) in (iii). The change of notation will be justified at once.
‡ If (A′) is true, we could choose for I the value of ![]() and for n any integer greater than
and for n any integer greater than ![]() since the solution then has the minimizing property.
since the solution then has the minimizing property.
* Compare M. Morse, loc. cit. p. 228.
* Where n is chosen according to footnote ‡ on p. 90.
† It will be obvious by (19) and (7), that this set is stationary for large N and it could be made stationary for any N by a proper choice of I.
* Loc. cit. p. 271
† Loc. cit. p. 258.