Linear forms in algebraic points of Abelian functions. I
Published online by Cambridge University Press: 24 October 2008
Extract
Let Ω be a Riemann matrix whose 2n columns are vectors of Cn. It is well-known (e.g. (10)) that the field 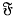 of meromorphic functions on Cn with these vectors among their periods is of transcendence degree n over C. More precisely, this field can be written as C(A, B) where A = (A1, …, An) is a vector of algebraically independent functions of the variable z = (z1, …, zn) and B is algebraic over C(A). We shall assume that B is in fact integral of degree d over the ring C[A]. Since the derivatives ∂f/∂zi of a periodic function f(z) are also periodic, the field
of meromorphic functions on Cn with these vectors among their periods is of transcendence degree n over C. More precisely, this field can be written as C(A, B) where A = (A1, …, An) is a vector of algebraically independent functions of the variable z = (z1, …, zn) and B is algebraic over C(A). We shall assume that B is in fact integral of degree d over the ring C[A]. Since the derivatives ∂f/∂zi of a periodic function f(z) are also periodic, the field 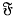 is mapped into itself by the differential operators ∂/∂zi. Thus there exists a function C(z) in C[A] such that these operators map the ring C[A, B, C−1] into itself.
is mapped into itself by the differential operators ∂/∂zi. Thus there exists a function C(z) in C[A] such that these operators map the ring C[A, B, C−1] into itself.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 77 , Issue 3 , May 1975 , pp. 499 - 513
- Copyright
- Copyright © Cambridge Philosophical Society 1975
References
REFERENCES
- 9
- Cited by


