A note on lemmas of Green and Kondo
Published online by Cambridge University Press: 24 October 2008
Extract
Let R be the field of rational numbers, {x} = {x1, z2, …}, {y} = {y1, y2, …} be two countably infinite sets of variables and t an indeterminate. Let (λ) = (λ1, λ2, …, λm) be a partition of n. Then Littlewood (5) has shown that
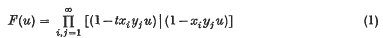
can be expressed in the form
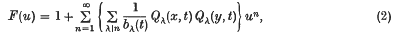
where Qλ(x, t) and Qλ(y, t) denote certain symmetric functions on the sets {x} and {y} respectively. In addition
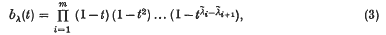
where  is the partition of n conjugate to (λ). In fact, Littlewood (5) showed that
is the partition of n conjugate to (λ). In fact, Littlewood (5) showed that
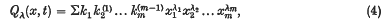
where the summation is over all terms obtained by permutations of the variables xi (i = 1, 2, …) and
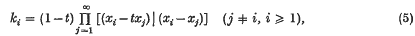
 .
.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 63 , Issue 1 , January 1967 , pp. 83 - 85
- Copyright
- Copyright © Cambridge Philosophical Society 1967
References
REFERENCES
- 1
- Cited by


