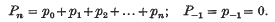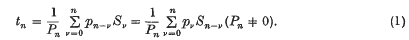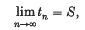Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Izumi, Masako
and
Izumi, Shin-ichi
1970.
Absolute Nörlund summability of Fourier series of functions of bounded variation.
Bulletin of the Australian Mathematical Society,
Vol. 3,
Issue. 1,
p.
111.
Ram, R. D.
and
Lal, Shiva N.
1972.
On the behaviour of a series associated with the allied series of a Fourier series.
Kodai Mathematical Journal,
Vol. 24,
Issue. 2,
Jena, Subas Chandra
and
Swain, Sakuntala
1989.
Generalized absolute Cesaro summability of a Fourier series.
Proceedings of the Indian Academy of Sciences - Section A,
Vol. 99,
Issue. 2,
p.
163.
Das, G.
and
Ray, B. K.
2009.
Lack of Tauberian theorem for absolute almost convergence.
Analysis Mathematica,
Vol. 35,
Issue. 1,
p.
37.