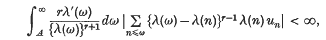Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ray, B. K.
1970.
On the absolute summability of some series related to a Fourier series.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 67,
Issue. 1,
p.
29.


 is summable |R, λ, r|, where r > 0, if
is summable |R, λ, r|, where r > 0, if