Rigid homogeneous chains
Published online by Cambridge University Press: 24 October 2008
Extract
Classifying (unordered) sets by the elementary (first order) properties of their automorphism groups was undertaken in (7), (9) and (11). For example, if Ω is a set whose automorphism group, S(Ω), satisfies
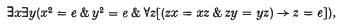
then Ω has cardinality at most ℵ0 and conversely (see (7)). We are interested in classifying homogeneous totally ordered sets (homogeneous chains, for short) by the elementary properties of their automorphism groups. (Note that we use ‘homogeneous’ here to mean that the automorphism group is transitive.) This study was begun in (4) and (5). For any set Ω, S(Ω) is primitive (i.e. has no congruences). However, the automorphism group of a homogeneous chain need not be o-primitive (i.e. it may have convex congruences). Fortunately, ‘o-primitive’ is a property that can be captured by a first order sentence for automorphisms of homogeneous chains. Hence our general problem falls naturally into two parts. The first is to classify (first order) the homogeneous chains whose automorphism groups are o-primitive; the second is to determine how the o-primitive components are related for arbitrary homogeneous chains whose automorphism groups are elementarily equivalent.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 89 , Issue 1 , January 1981 , pp. 07 - 17
- Copyright
- Copyright © Cambridge Philosophical Society 1981
References
- 11
- Cited by


