Published online by Cambridge University Press: 18 January 2011
We define the symmetric Auslander category As(R) to consist of complexes of projective modules whose left- and right-tails are equal to the left- and right-tails of totally acyclic complexes of projective modules.
The symmetric Auslander category contains A(R), the ordinary Auslander category. It is well known that A(R) is intimately related to Gorenstein projective modules, and our main result is that As(R) is similarly related to what can reasonably be called Gorenstein projective homomorphisms. Namely, there is an equivalence of triangulated categories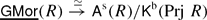 where GMor(R) is the stable category of Gorenstein projective objects in the abelian category Mor(R) of homomorphisms of R-modules.
where GMor(R) is the stable category of Gorenstein projective objects in the abelian category Mor(R) of homomorphisms of R-modules.
This result is set in the wider context of a theory for As(R) and Bs(R), the symmetric Bass category which is defined dually.