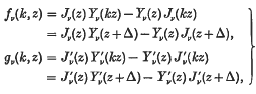Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Naylor, D.
and
Dennis, S. C. R.
1968.
On a singular eigenvalue problem.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 64,
Issue. 2,
p.
439.
Salchev, L. Z.
and
Popov, V. B.
1973.
Determination of the zeros of a cross-product Bessel function.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 74,
Issue. 3,
p.
477.
1975.
Mathematical Functions and their Approximations.
p.
517.
Salchev, L. Z.
and
Popov, V. B.
1976.
A Property of the Zeros of Cross‐Product Bessel Functions of Different Orders.
ZAMM - Journal of Applied Mathematics and Mechanics / Zeitschrift für Angewandte Mathematik und Mechanik,
Vol. 56,
Issue. 2-3,
p.
120.
Horvat, Martin
and
Prosen, Tomaž
2007.
The bends on a quantum waveguide and cross-products of Bessel functions.
Journal of Physics A: Mathematical and Theoretical,
Vol. 40,
Issue. 24,
p.
6349.
Sorolla, Eden
Mosig, Juan R.
and
Mattes, Michael
2013.
Algorithm to Calculate a Large Number of Roots of the Cross-Product of Bessel Functions.
IEEE Transactions on Antennas and Propagation,
Vol. 61,
Issue. 4,
p.
2180.
Ziener, Christian H
Kurz, Felix T
Buschle, Lukas R
and
Kampf, Thomas
2015.
Orthogonality, Lommel integrals and cross product zeros of linear combinations of Bessel functions.
SpringerPlus,
Vol. 4,
Issue. 1,
Budzinskiy, S.S.
Larichev, A.V.
and
Razgulin, A.V.
2018.
Reducing dimensionality to model 2D rotating and standing waves in a delayed nonlinear optical system with thin annulus aperture.
Nonlinear Analysis: Real World Applications,
Vol. 44,
Issue. ,
p.
559.
Budzinskiy, S. S.
and
Romanenko, T. E.
2020.
Fast Discrete Finite Hankel Transform for Equations in a Thin Annulus.
Computational Mathematics and Modeling,
Vol. 31,
Issue. 3,
p.
364.
Budzinskiy, S. S.
2020.
On the Zeros of Cross-Product Bessel Functions in Oblique Derivative Boundary-Value Problems.
Moscow University Computational Mathematics and Cybernetics,
Vol. 44,
Issue. 2,
p.
53.
Guo, Jingwei
Müller, Wolfgang
Wang, Weiwei
and
Wang, Zuoqin
2021.
The Weyl formula for planar annuli.
Journal of Functional Analysis,
Vol. 281,
Issue. 4,
p.
109063.
Olendski, O.
2022.
Quantum-information theory of a Dirichlet ring with Aharonov–Bohm field.
The European Physical Journal Plus,
Vol. 137,
Issue. 4,
Wang, Weiwei
2023.
A uniform bound on the multiplicity of Dirichlet eigenvalues in planar annuli.
Journal of Mathematical Analysis and Applications,
Vol. 518,
Issue. 1,
p.
126616.