Published online by Cambridge University Press: 24 October 2008
In this paper we prove several results concerning the complexity of a set relative to compact sets. We prove that for any Polish space X and Borel set B ⊆ X, if B is not 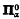 , then there exists a compact zero-dimensional P ⊆ X such that p ∩ X is not
, then there exists a compact zero-dimensional P ⊆ X such that p ∩ X is not 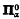 . We also show that it is consistent with ZFC that, for any A ⊆ ωω, if for all compact K ⊆ ωωA ∩ K is
. We also show that it is consistent with ZFC that, for any A ⊆ ωω, if for all compact K ⊆ ωωA ∩ K is 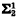 , then A is
, then A is 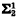 . This generalizes to
. This generalizes to  in place of
in place of 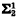 assuming the consistency of some hypotheses involving determinacy. We give an alternative proof of the following theorem of Saint-Raymond. Suppose X and Y are compact metric spaces and f is a continuous surjection of X onto Y. Then, for any A ⊆ Y, A is
assuming the consistency of some hypotheses involving determinacy. We give an alternative proof of the following theorem of Saint-Raymond. Suppose X and Y are compact metric spaces and f is a continuous surjection of X onto Y. Then, for any A ⊆ Y, A is 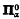 in Y iff f−1(A) is
in Y iff f−1(A) is 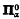 in X. The non-trivial part of this result is to show that taking pre-images cannot reduce the Borel complexity of a set. The techniques we use are the definability of forcing and Wadge games.
in X. The non-trivial part of this result is to show that taking pre-images cannot reduce the Borel complexity of a set. The techniques we use are the definability of forcing and Wadge games.