Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Stretch, C. T.
1981.
Stable cohomotopy and cobordism of abelian groups.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 90,
Issue. 2,
p.
273.
1982.
Vector Bundles.
Vol. 101,
Issue. ,
p.
303.
Vallejo, Ernesto
1990.
Polynomial operations on stable cohomotopy.
Manuscripta Mathematica,
Vol. 67,
Issue. 1,
p.
345.
Vallejo, Ernesto
1990.
Polynomial operations from burnside rings to representation functors.
Journal of Pure and Applied Algebra,
Vol. 65,
Issue. 2,
p.
163.
Hunton, J.R.
and
Schuster, B.
2005.
Subalgebras of group cohomology defined by infinite loop spaces.
Topology,
Vol. 44,
Issue. 4,
p.
689.
La Vecchia, Marco
2024.
The local (co)homology theorems for equivariant bordism.
Geometry & Topology,
Vol. 28,
Issue. 2,
p.
627.


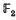 ). (
). (
