Published online by Cambridge University Press: 24 October 2008
Compact right topological groups appear naturally in topological dynamics. Some continuity properties of the one arising as an enveloping semigroup from the distal function 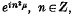 are considered here (and, by way of comparison, the enveloping semigroups arising from two almost automorphic functions are discussed). The continuity properties are established either explicitly or by citing a theorem which is proved here and gives some characterizations of almost periodic functions. One characterization is proved using the result (essentially due to W. A. Veech) that a distal, almost automorphic function is almost periodic. A proof of this last result is also given.
are considered here (and, by way of comparison, the enveloping semigroups arising from two almost automorphic functions are discussed). The continuity properties are established either explicitly or by citing a theorem which is proved here and gives some characterizations of almost periodic functions. One characterization is proved using the result (essentially due to W. A. Veech) that a distal, almost automorphic function is almost periodic. A proof of this last result is also given.