Hostname: page-component-6bb9c88b65-dwch4 Total loading time: 0 Render date: 2025-07-20T05:57:06.820Z Has data issue: false hasContentIssue false
  • English
  • Français

The evaluation of integrals of the form

Published online by Cambridge University Press:  24 October 2008

E. T. Goodwin
E. T. Goodwin
Affiliation:
Mathematics Division, National Physical Laboratory, Teddington, Middlesex
Get access Rights & Permissions [Opens in a new window]

Extract

It is well known to computers that the approximate formula

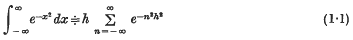
yields a surprising degree of accuracy even for quite large values of the interval h; for example, if h = 1 the error is one unit in the fourth decimal.

Information

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 45 , Issue 2 , April 1949 , pp. 241 - 245
Copyright
Copyright © Cambridge Philosophical Society 1949

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

* See, for example, Milne-Thomson, L. M., The calculus of finite differences (Macmillan, 1933).Google Scholar

* Turing, A. M., ‘A method for the calculation of the zeta-function’, Proc. London Math. Soc. (2), 48 (1943), 180.Google Scholar