No CrossRef data available.
A family of elliptic curves and cyclic cubic field extensions*
Published online by Cambridge University Press: 24 October 2008
Extract
Let K be a field with char K ≡ 2,3. We consider the problem of finding rational points over K on the family of elliptic curves Fλ, given in homogeneous coordinates (over 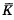 ) by
) by

- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 96 , Issue 1 , July 1984 , pp. 39 - 43
- Copyright
- Copyright © Cambridge Philosophical Society 1984


