Article contents
Finite group schemes of p-rank ≤ 1
Published online by Cambridge University Press: 27 November 2017
Abstract
Let 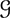 be a finite group scheme over an algebraically closed field k of characteristic char(k) = p ≥ 3. In generalisation of the familiar notion from the modular representation theory of finite groups, we define the p-rank rkp(
be a finite group scheme over an algebraically closed field k of characteristic char(k) = p ≥ 3. In generalisation of the familiar notion from the modular representation theory of finite groups, we define the p-rank rkp(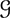 ) of
) of 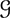 and determine the structure of those group schemes of p-rank 1, whose linearly reductive radical is trivial. The most difficult case concerns infinitesimal groups of height 1, which correspond to restricted Lie algebras. Our results show that group schemes of p-rank ≤ 1 are closely related to those being of finite or domestic representation type.
and determine the structure of those group schemes of p-rank 1, whose linearly reductive radical is trivial. The most difficult case concerns infinitesimal groups of height 1, which correspond to restricted Lie algebras. Our results show that group schemes of p-rank ≤ 1 are closely related to those being of finite or domestic representation type.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 166 , Issue 2 , March 2019 , pp. 297 - 323
- Copyright
- Copyright © Cambridge Philosophical Society 2017
References
REFERENCES
- 3
- Cited by


