Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Roe, John
1988.
An index theorem on open manifolds. II.
Journal of Differential Geometry,
Vol. 27,
Issue. 1,
Heitsch, James L.
and
Lazarov, Connor
1990.
A Lefschetz theorem for foliated manifolds.
Topology,
Vol. 29,
Issue. 2,
p.
127.
Douglas, R. G.
Hurder, S.
and
Kaminker, J.
1991.
Cyclic cocycles, renormalization and eta-invariants.
Inventiones Mathematicae,
Vol. 103,
Issue. 1,
p.
101.
Glazebrook, J. F.
and
Kamber, F. W.
1991.
Transversal Dirac families in Riemannian foliations.
Communications in Mathematical Physics,
Vol. 140,
Issue. 2,
p.
217.
Ramachandran, Mohan
1993.
von Neumann index theorems for manifolds with boundary.
Journal of Differential Geometry,
Vol. 38,
Issue. 2,
Douglas, Ronald G.
Glazebrook, James F.
Kamber, Franz W.
and
Yu, Guoliang
1995.
Index formulas for geometric Dirac operators in Riemannian foliations.
K-Theory,
Vol. 9,
Issue. 5,
p.
407.
Heitsch, James L.
1995.
Bismut superconnections and the Chern character for Dirac operators on foliated manifolds.
K-Theory,
Vol. 9,
Issue. 6,
p.
507.
Douglas, Ronald G.
and
Kaminker, Jerome
1997.
Induced Cyclic Cocycles and Higher Eta Invariants.
Journal of Functional Analysis,
Vol. 147,
Issue. 2,
p.
301.
Ceccherini-Silberstein, T.G.
and
Samet-Vaillant, A.Y.
2008.
Gromov's translation algebras, growth and amenability of operator algebras.
Expositiones Mathematicae,
Vol. 26,
Issue. 2,
p.
141.
Álvarez López, Jesús A.
and
Kordyukov, Yuri A.
2008.
C*-algebras and Elliptic Theory II.
p.
1.
Sanguiao, Luis
2008.
L 2-invariants of Riemannian foliations.
Annals of Global Analysis and Geometry,
Vol. 33,
Issue. 3,
p.
271.
Ceccherini-Silberstein, T. G.
and
Samet-Vaillant, A. Y.
2009.
Asymptotic invariants of finitely generated algebras. A generalization of Gromov's quasi-isometric viewpoint.
Journal of Mathematical Sciences,
Vol. 156,
Issue. 1,
p.
56.
Bartoszek, Adam
Kalina, Jerzy
and
Pierzchalski, Antoni
2011.
Gradients for SL(q)-foliations.
Journal of Geometry and Physics,
Vol. 61,
Issue. 12,
p.
2410.
Sande, L. Sanguiao
2011.
L 2-Lefschetz distribution of Lie foliations.
Geometriae Dedicata,
Vol. 151,
Issue. 1,
p.
305.
Moriyoshi, Hitoshi
and
Piazza, Paolo
2012.
Eta Cocycles, Relative Pairings and the Godbillon–Vey Index Theorem.
Geometric and Functional Analysis,
Vol. 22,
Issue. 6,
p.
1708.
Slesar, Vladimir
2012.
A vanishing result for the Spin c Dirac operator defined along the leaves of a foliation.
Geometriae Dedicata,
Vol. 161,
Issue. 1,
p.
239.
Antonini, Paolo
2013.
The Atiyah–Patodi–Singer index formula for measured foliations.
Bulletin des Sciences Mathématiques,
Vol. 137,
Issue. 2,
p.
140.
Yuncken, Robert
2013.
FoliationC⁎-algebras on multiply fibred manifolds.
Journal of Functional Analysis,
Vol. 265,
Issue. 9,
p.
1829.
Bohlen, Karsten
and
Schrohe, Elmar
2018.
Getzler rescaling via adiabatic deformation and a renormalized index formula.
Journal de Mathématiques Pures et Appliquées,
Vol. 120,
Issue. ,
p.
220.
Park, Efton
2020.
Operator Theory, Operator Algebras and Their Interactions with Geometry and Topology.
Vol. 278,
Issue. ,
p.
455.
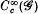 associated to a foliation ℱ of the compact manifold M. Here
associated to a foliation ℱ of the compact manifold M. Here 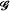 is the graph or holonomy groupoid of the foliation ℱ (Winkelnkemper [15]). By forming the completion of
is the graph or holonomy groupoid of the foliation ℱ (Winkelnkemper [15]). By forming the completion of 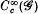 in its regular representation, he obtains the C*-algebra C*{M, ℱ) associated to the foliation. The completeness of C*(M, ℱ) makes it easier to handle in some analytical contexts, but in others it seems to be too big, and it is necessary to consider instead some carefully selected dense subalgebra (cf. [6]). The purpose of this note is to show that certain spectral functions of leafwise elliptic operators, which might a priori be expected to belong to C*(M, ℱ), in fact belong to the more controllable dense subalgebra
in its regular representation, he obtains the C*-algebra C*{M, ℱ) associated to the foliation. The completeness of C*(M, ℱ) makes it easier to handle in some analytical contexts, but in others it seems to be too big, and it is necessary to consider instead some carefully selected dense subalgebra (cf. [6]). The purpose of this note is to show that certain spectral functions of leafwise elliptic operators, which might a priori be expected to belong to C*(M, ℱ), in fact belong to the more controllable dense subalgebra 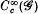 . We give a couple of applications, including a proof not passing through C*-algebras of Connes' index theorem for measured foliations [4]. It should be emphasized that the proof of that result offered here is essentially Connes' one, but the presentation may perhaps be more congenial to those who are not C*-algebra specialists.
. We give a couple of applications, including a proof not passing through C*-algebras of Connes' index theorem for measured foliations [4]. It should be emphasized that the proof of that result offered here is essentially Connes' one, but the presentation may perhaps be more congenial to those who are not C*-algebra specialists.

