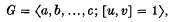Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Allenby, R. B. J. T.
and
Tang, C. Y.
1985.
Residual finiteness of certain 1-relator groups: extensions of results of Gilbert Baumslag.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 97,
Issue. 2,
p.
225.
Loginova, E. D.
1999.
Residual finiteness of the free product of two groups with commuting subgroups.
Siberian Mathematical Journal,
Vol. 40,
Issue. 2,
p.
341.
BIRMAN, JOAN S.
RAMPICHINI, MARTA
BOLDI, PAOLO
and
VIGNA, SEBASTIANO
2002.
TOWARDS AN IMPLEMENTATION OF THE B–H ALGORITHM FOR RECOGNIZING THE UNKNOT.
Journal of Knot Theory and Its Ramifications,
Vol. 11,
Issue. 04,
p.
601.
Tieudjo, D.
and
Moldavanskii, D. I.
2006.
On the Automorphisms of Some One-Relator Groups.
Communications in Algebra,
Vol. 34,
Issue. 11,
p.
3975.
BAUMSLAG, GILBERT
FINE, BENJAMIN
MILLER, CHARLES F.
and
TROEGER, DOUGLAS
2009.
VIRTUAL PROPERTIES OF CYCLICALLY PINCHED ONE-RELATOR GROUPS.
International Journal of Algebra and Computation,
Vol. 19,
Issue. 02,
p.
213.
Tieudjo, D.
Moldavanskii, D. I.
and
Bell, Howard
2010.
Conjugacy Separability of Some One‐Relator Groups.
International Journal of Mathematics and Mathematical Sciences,
Vol. 2010,
Issue. 1,
Martínez-Pérez, Conchita
2014.
Finite presentability of normal fibre products.
Journal of Pure and Applied Algebra,
Vol. 218,
Issue. 8,
p.
1373.
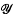 -group. This class
-group. This class  contains, in particular, the class X of those groups G which can be presented in the form
contains, in particular, the class X of those groups G which can be presented in the form