Further aspects of the evaluation of σ(m. n≠0, 0) (am2 + bnm + cn2)−s
Published online by Cambridge University Press: 24 October 2008
Extract
In some previous publications, Zucker and Robertson [13, 14, 15] for certain cases evaluated exactly the double sum
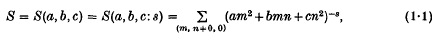
with a, b, c integers. In (1·1) the summation is over all integer values of m and n, both positive and negative, but excluding the case where m and n are simultaneously zero. The term ‘exact’ used here is in the sense introduced by Glasser [5], and means that S may be expressed as a linear sum of products of pairs of Dirichlet L-series. S is then said to be solvable. Whether S may be solved or not depends on the properties of the related binary quadratic form (am2 + bmn + cn2) = (a, b, c). The cases considered in [15] were when a > 0 and d = b2 − 4ac < 0, i.e. (a, b, c) was positive definite. When this is so, Glasser [5] conjectured that S was solvable if and only if (a, b, c) had one reduced form per genus, i.e. the reduced forms of (a, b, c) were disjoint. Zucker and Robertson [15] were in fact able to solve S for all (a, b, c) for which d < 0 and whose reduced forms were disjoint. A complete list of solutions may be found in [6].
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 95 , Issue 1 , January 1984 , pp. 5 - 13
- Copyright
- Copyright © Cambridge Philosophical Society 1984
References
REFERENCES
- 10
- Cited by


