 1-groups
1-groupsPublished online by Cambridge University Press: 24 October 2008
Following Robinson(4), we shall use the term Abelian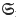 1-group to describe an Abelian group A of finite (Mal'cev special or Prüfer) rank, whose torsion subgroup T is Černikov. These groups were called Abelian groups of type A3 by Mal'cev (3). Let G = Aut A. It is not hard to see that G/CG(A/T) and G/CG(T) are linear groups, and CG(A/T) ∩ CG(T) is Abelian. We improve on this observation by proving
1-group to describe an Abelian group A of finite (Mal'cev special or Prüfer) rank, whose torsion subgroup T is Černikov. These groups were called Abelian groups of type A3 by Mal'cev (3). Let G = Aut A. It is not hard to see that G/CG(A/T) and G/CG(T) are linear groups, and CG(A/T) ∩ CG(T) is Abelian. We improve on this observation by proving
Theorem 1. The group G contains a normal Abelian Černikov subgroup G0, such that G/G0is linear over a field of characteristic zero.