Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T05:28:02.869Z Has data issue: false hasContentIssue false
  • English
  • Français

The Hausdorff dimension of self-affine fractals

Published online by Cambridge University Press:  24 October 2008

K. J. Falconer
K. J. Falconer
Affiliation:
School of Mathematics, University Walk, Bristol BS8 1TW, England
Get access Rights & Permissions [Opens in a new window]

Abstract

If T is a linear transformation on ℝn with singular values α1 ≥ α2 ≥ … ≥ αn, the singular value function øs is defined by where m is the smallest integer greater than or equal to s. Let T1, …, Tk be contractive linear transformations on ℝn. Let where the sum is over all finite sequences (i1, …, ir) with 1 ≤ ij ≤ k. Then for almost all (a1, …, ak) ∈ ℝnk, the unique non-empty compact set F satisfying 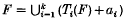has Hausdorff dimension min{d, n}. Moreover the ‘box counting’ dimension of F is almost surely equal to this number.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 103 , Issue 2 , March 1988 , pp. 339 - 350
Copyright
Copyright © Cambridge Philosophical Society 1988

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bedford, T.. Dimension and dynamics for fractal recurrent sets. J. London Math. Soc. (2) 33 (1986), 89–100.Google Scholar
[2]Bedford, T.. Ph.D. thesis, University of Warwick (1984).Google Scholar
[3]Besicovitch, A. S.. On the existence of subsets of finite measure of sets of infinite measure. Indag. Math. 14 (1952), 339–344.CrossRefGoogle Scholar
[4]Falconer, K. J.. The Geometry of Fractal Sets (Cambridge University Press, 1985).CrossRefGoogle Scholar
[5]Falconer, K. J.. The Hausdorff dimension of some fractals and attractors of overlapping construction. J. Statist. Phys. 47 (1987), 123–132.CrossRefGoogle Scholar
[6]Furstenberg, H.. Non-commuting random products. Trans. Amer. Math. Soc. 108 (1963), 377–428.CrossRefGoogle Scholar
[7]Furstenberg, H. and Kesten, H.. Products of random matrices. Ann. Math. Statistics 31 (1960), 457–469.CrossRefGoogle Scholar
[8]Geronimo, J. S. and Hardin, D. P.. An exact formula for the measure dimensions associated with a class of piecewise linear maps (to appear).Google Scholar
[9]Hutchinson, J. E.. Fractals and self-similarity. Indiana Univ. Math. J. 30 (1981), 713–747.CrossRefGoogle Scholar
[10]Kingman, J. F. C.. Subadditive processes. In École d'Été de Probabilitiés de Saint-Flour V, Lecture Notes in Math. vol. 539 (Springer-Verlag, 1976). pp. 167–223.Google Scholar
[11]Mattila, P.. Hausdorff dimension, orthogonal projections and intersections with planes. Ann. Acad. Sci. Fenn. Ser. A I Math. 1 (1975), 227–244.CrossRefGoogle Scholar
[12]McMullen, C.. The Hausdorff dimension of general Sierpinśki carpets. Nagoya Math. J. 96 (1984), 1–9.CrossRefGoogle Scholar
[13]Moran, P. A. P.. Additive functions of intervals and Hausdorff measure. Proc. Cambridge Philos. Soc. 42 (1946), 15–23.Google Scholar
[14]Rogers, C. A.. Hausdorff Measures (Cambridge University Press, 1970).Google Scholar
[15]Ruelle, D.. Bowen's formula for the dimension of self-similar sets. In Progress in Physics 7, (Birkhauser-Verlag, 1983). pp. 351–357.Google Scholar