Homological finiteness conditions for modules over strongly group-graded rings
Published online by Cambridge University Press: 24 October 2008
Extract
Throughout this paper, k denotes a commutative ring. We will develop a theory of homological finiteness conditions for modules over certain graded k-algebras which generalizes known theory for group algebras. The simplest of our results, Theorem A below, generalizes certain results of Aljadeff and Yi on crossed products of polycyclic-by-finite groups (cf. [1, 11]), but also applies to many other crossed products in cases where little was previously known. Before stating the results, we recall definitions of graded and strongly graded rings. Let G be a monoid. Naively, a G-graded k-algebra is a k-algebra R which admits a k-module decomposition,

in such a way that Rg Rh ⊆ for all g, h ∈ G. If R is a G-graded k-algebra and X is any subset of G, then we write Rx for the k-submodule of R supported on X; that is
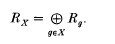
Note that if H is a submonoid of G then RH is a subalgebra of R.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 120 , Issue 1 , July 1996 , pp. 43 - 54
- Copyright
- Copyright © Cambridge Philosophical Society 1996
References
REFERENCES
- 12
- Cited by


