Published online by Cambridge University Press: 01 March 2008
Let Sj: ℝd → ℝd for j = 1, . . ., N be contracting similarities. Also, let (p1,. . ., pN, p) be a probability vector and let ν be a probability measure on ℝd with compact support. We show that there exists a unique probability measure μ such that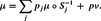 The measure μ is called an in-homogenous self-similar measure. In this paper we study the asymptotic behaviour of the Fourier transforms of in-homogenous self-similar measures. Finally, we present a number of applications of our results. In particular, non-linear self-similar measures introduced and investigated by Glickenstein and Strichartz are special cases of in-homogenous self-similar measures, and as an application of our main results we obtain simple proofs of generalizations of Glickenstein and Strichartz's results on the asymptotic behaviour of the Fourier transforms of non-linear self-similar measures.
The measure μ is called an in-homogenous self-similar measure. In this paper we study the asymptotic behaviour of the Fourier transforms of in-homogenous self-similar measures. Finally, we present a number of applications of our results. In particular, non-linear self-similar measures introduced and investigated by Glickenstein and Strichartz are special cases of in-homogenous self-similar measures, and as an application of our main results we obtain simple proofs of generalizations of Glickenstein and Strichartz's results on the asymptotic behaviour of the Fourier transforms of non-linear self-similar measures.