No CrossRef data available.
Published online by Cambridge University Press: 24 October 2008
Let nℕ{1} and let S1, , Sn be isometries on an infinite-dimensional Hilbert space such that 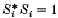 for each i and
for each i and 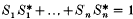 . It was shown in 1 that the C*-algebra On generated by S1, , Sn is an infinite simple C*-algebra which is, up to isomorphism, independent of the choice of isometries satisfying the given relations. Ifis a unital *-endomorphism of On then, as shown in 2,
. It was shown in 1 that the C*-algebra On generated by S1, , Sn is an infinite simple C*-algebra which is, up to isomorphism, independent of the choice of isometries satisfying the given relations. Ifis a unital *-endomorphism of On then, as shown in 2, 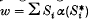 is a unitary determiningby the equations (Si) = w*Si and each unitary arises in this way.
is a unitary determiningby the equations (Si) = w*Si and each unitary arises in this way.