Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
1987.
Saks Spaces and Applications to Functional Analysis.
Vol. 139,
Issue. ,
p.
279.
1987.
Saks Spaces and Applications to Functional Analysis.
Vol. 139,
Issue. ,
p.
1.
Chilin, Vladimir I.
Medzhitov, Arthur M.
and
Sukochev, Pheodor A.
1989.
Isometries of non-commutative Lorentz spaces.
Mathematische Zeitschrift,
Vol. 200,
Issue. 4,
p.
527.
Ayupov, Sh. A.
and
Abdullaev, R. Z.
1989.
Quantum Probability and Applications IV.
Vol. 1396,
Issue. ,
p.
99.
Ferleger, Sergei V.
and
Sukochev, Fyodor A.
1996.
On the contractibility to a point of the linear groups of reflexive non-commutative Lp-spaces.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 119,
Issue. 3,
p.
545.
Sukochev, F. A.
1996.
Isometries of symmetric operator spaces associated with AFD factors of typeII and symmetric vector-valued spaces.
Integral Equations and Operator Theory,
Vol. 26,
Issue. 1,
p.
102.
Sukochev, F.
1996.
Non-isomorphism of 𝐿_{𝑝}-spaces associated with finite and infinite von Neumann algebras.
Proceedings of the American Mathematical Society,
Vol. 124,
Issue. 5,
p.
1517.
Sukochev, F. A.
2000.
Linear-topological classification of separableL p-spaces associated with von Neumann algebras of type I.
Israel Journal of Mathematics,
Vol. 115,
Issue. 1,
p.
137.
Antoniou, I.
Majewski, W. A.
and
Suchanecki, Z.
2002.
Implementability of Liouville Evolution, Koopman and Banach-Lamperti Theorems in Classical and Quantum Dynamics.
Open Systems & Information Dynamics,
Vol. 09,
Issue. 04,
p.
301.
Ng, Ping Wong
and
Ozawa, Narutaka
2002.
A characterization of completely 1-complemented subspaces of noncommutative L1-spaces.
Pacific Journal of Mathematics,
Vol. 205,
Issue. 1,
p.
171.
Majewski, Władysław A.
2004.
On Positive Maps, Entanglement and Quantization.
Open Systems & Information Dynamics,
Vol. 11,
Issue. 01,
p.
43.
Sherman, David
2005.
Noncommutative Lp structure encodes exactly Jordan structure.
Journal of Functional Analysis,
Vol. 221,
Issue. 1,
p.
150.
Junge, Marius
Ruan, Zhong-Jin
and
Sherman, David
2005.
A classification for 2-isometries of noncommutativeL p-spaces.
Israel Journal of Mathematics,
Vol. 150,
Issue. 1,
p.
285.
Junge, Marius
and
Le Merdy, Christian
2007.
Dilations and rigid factorisations on noncommutative Lp-spaces.
Journal of Functional Analysis,
Vol. 249,
Issue. 1,
p.
220.
He, Kan
and
Yuan, Qing
2014.
Nonlinear Isometries on Schatten-pClass in Atomic Nest Algebras.
Abstract and Applied Analysis,
Vol. 2014,
Issue. ,
p.
1.
Bekka, Bachir
2015.
Local rigidity for actions of Kazhdan groups on noncommutative Lp-spaces.
International Journal of Mathematics,
Vol. 26,
Issue. 08,
p.
1550064.
Li, Yuan
and
Li, Yu-E
2015.
Some characterizations of the trace norm triangle equality.
Linear Algebra and its Applications,
Vol. 484,
Issue. ,
p.
396.
Leung, Chi-Wai
Ng, Chi-Keung
and
Wong, Ngai-Ching
2017.
The positive contractive part of a noncommutative L-space is a complete Jordan invariant.
Linear Algebra and its Applications,
Vol. 519,
Issue. ,
p.
102.
Sukochev, F.
and
Veksler, A.
2018.
Positive Linear Isometries in Symmetric Operator Spaces.
Integral Equations and Operator Theory,
Vol. 90,
Issue. 5,
Ray, Samya Kumar
2020.
On isometric embedding ℓpm→S∞ and unique operator space structure.
Bulletin of the London Mathematical Society,
Vol. 52,
Issue. 3,
p.
437.
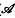 , φ) for 1 ≤ p ≤ ∞, where φ is a faithful semifinite normal trace on a von Neumann algebra
, φ) for 1 ≤ p ≤ ∞, where φ is a faithful semifinite normal trace on a von Neumann algebra 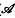 , are defined in (10),(2),(14). The problem of determining the general form of an isometry
, are defined in (10),(2),(14). The problem of determining the general form of an isometry 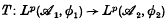 of one such space into another has been studied in (i), (6), (9), (12), (5). Our main result, Theorem 2, is a characterization of such isometries for 1 ≤ p ≤ ∞, ≠ 2. The method of proof is based on that of (7), where isometries between Lp function spaces are characterized. The main step in the proof is Theorem 1, which gives the conditions under which equality holds in Clarkson's inequality.
of one such space into another has been studied in (i), (6), (9), (12), (5). Our main result, Theorem 2, is a characterization of such isometries for 1 ≤ p ≤ ∞, ≠ 2. The method of proof is based on that of (7), where isometries between Lp function spaces are characterized. The main step in the proof is Theorem 1, which gives the conditions under which equality holds in Clarkson's inequality.

