Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Michelacakis, Nickolas
2005.
On a special class of fibrations and Kähler rigidity.
Pacific Journal of Mathematics,
Vol. 219,
Issue. 2,
p.
311.
Dekimpe, K.
Sadowski, M.
and
Szczepański, A.
2006.
Spin Structures on Flat Manifolds.
Monatshefte für Mathematik,
Vol. 148,
Issue. 4,
p.
283.
Miatello, Roberto
and
Podestá, Ricardo
2006.
The spectrum of twisted Dirac operators on compact flat manifolds.
Transactions of the American Mathematical Society,
Vol. 358,
Issue. 10,
p.
4569.
DEKIMPE, Karel
HAŁENDA, Marek
and
SZCZEPAŃSKI, Andrzej
2009.
Kähler flat manifolds.
Journal of the Mathematical Society of Japan,
Vol. 61,
Issue. 2,
Marin, Ivan
2016.
Crystallographic groups and flat manifolds from complex reflection groups.
Geometriae Dedicata,
Vol. 182,
Issue. 1,
p.
233.
Hałenda, Marek
2017.
Complex Hantzsche-Wendt manifolds.
Geometriae Dedicata,
Vol. 187,
Issue. 1,
p.
1.
Ocampo, Oscar
and
Rodríguez-Nieto, José Gregorio
2019.
On Bieberbach subgroups of B/[P,P] and flat manifolds with cyclic holonomy Z2d.
Topology and its Applications,
Vol. 265,
Issue. ,
p.
106827.
Johnson, Francis E. A.
2019.
A flat projective variety with $D_8$-holonomy.
Tohoku Mathematical Journal,
Vol. 71,
Issue. 2,
HAMILTON, M. J. D.
and
KOTSCHICK, D.
2021.
Lagrangian foliations and Anosov symplectomorphisms on Kähler manifolds.
Ergodic Theory and Dynamical Systems,
Vol. 41,
Issue. 11,
p.
3325.
Lima Gonçalves, Daciberg
Guaschi, John
Ocampo, Oscar
and
de Miranda e Pereiro, Carolina
2021.
Crystallographic groups and flat manifolds from surface braid groups.
Topology and its Applications,
Vol. 293,
Issue. ,
p.
107560.
Ocampo, Oscar
2021.
Bieberbach groups and flat manifolds with finite abelian holonomy from Artin braid groups.
Journal of Knot Theory and Its Ramifications,
Vol. 30,
Issue. 09,
Cerqueira Dos Santos Júnior, Paulo Cesar
and
Ocampo, Oscar
2023.
Virtual braid groups, virtual twin groups and crystallographic groups.
Journal of Algebra,
Vol. 632,
Issue. ,
p.
567.
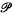 of fundamental groups of non-singular complex projective varieties is an interesting, but as yet imperfectly understood, class of finitely presented groups. Membership of
of fundamental groups of non-singular complex projective varieties is an interesting, but as yet imperfectly understood, class of finitely presented groups. Membership of 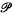 is known to be extremely restricted (see [22, 23]). In this paper, we employ geometrical rigidity properties to realize some group extensions as elements of
is known to be extremely restricted (see [22, 23]). In this paper, we employ geometrical rigidity properties to realize some group extensions as elements of 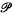 as in our previous papers, we find it convenient to work simultaneously with the class ℋ of fundamental groups of compact Kähler manifolds.
as in our previous papers, we find it convenient to work simultaneously with the class ℋ of fundamental groups of compact Kähler manifolds.

