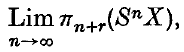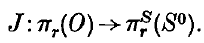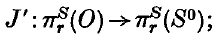Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
L�ffler, Peter
and
Smith, Larry
1974.
Line bundles over framed manifolds.
Mathematische Zeitschrift,
Vol. 138,
Issue. 1,
p.
35.
Kahn, Daniel S.
and
Priddy, Stewart B.
1978.
The transfer and stable homotopy theory.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 83,
Issue. 1,
p.
103.
Priddy, Stewart
1978.
Homotopy splittings involvingG andG/O.
Commentarii Mathematici Helvetici,
Vol. 53,
Issue. 1,
p.
470.
Snaith, Victor
1978.
On the stable cohomotopy of 𝑅𝑃^{∞}.
Proceedings of the American Mathematical Society,
Vol. 69,
Issue. 1,
p.
174.
Hoffman, P.
and
Zabrodsky, A.
1978.
Thin Lens Spaces.
Canadian Mathematical Bulletin,
Vol. 21,
Issue. 1,
p.
31.
May, J. P.
1979.
Algebraic Topology Aarhus 1978.
Vol. 763,
Issue. ,
p.
38.
Gray, Brayton
1984.
Unstable families related to the image of J.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 96,
Issue. 1,
p.
95.
Caruso, J.
Cohen, F. R.
May, J. P.
and
Taylor, L. R.
1984.
James maps, Segal maps, and the Kahn-Priddy theorem.
Transactions of the American Mathematical Society,
Vol. 281,
Issue. 1,
p.
243.
Micha, E.
1985.
Cobordism of (𝑘)-framed manifolds.
Transactions of the American Mathematical Society,
Vol. 291,
Issue. 1,
p.
269.
1986.
Vol. 121,
Issue. ,
p.
395.
Campbell, H. E. A.
Cohen, F. R.
Peterson, F. P.
and
Selick, P. S.
1986.
Self-maps of loop spaces. II.
Transactions of the American Mathematical Society,
Vol. 293,
Issue. 1,
p.
41.
Kuhn, Nicholas J.
1987.
Algebraic Topology.
Vol. 1286,
Issue. ,
p.
286.
Davis, Donald M.
and
Mahowald, Mark
1989.
The image of the stable J-homomorphism.
Topology,
Vol. 28,
Issue. 1,
p.
39.
Davis, Donald M.
and
Mahowald, Mark
1990.
v 1-Periodicity in the unstable adams spectral sequence.
Mathematische Zeitschrift,
Vol. 204,
Issue. 1,
p.
319.
Davis, Donald M.
and
Mahowald, Mark
1993.
υ1-Localizations of finite torsion spectra and spherically resolved spaces.
Topology,
Vol. 32,
Issue. 3,
p.
543.
Ахметьев, Петр Михайлович
and
Akhmet'ev, Petr Mikhailovich
2000.
Вложения компактов, стабильные гомотопические группы сфер и теория особенностей.
Успехи математических наук,
Vol. 55,
Issue. 3,
p.
3.
Akhmet’ev, P. M.
2009.
Geometric approach to stable homotopy groups of spheres. The Adams–Hopf invariants.
Journal of Mathematical Sciences,
Vol. 159,
Issue. 6,
p.
753.