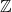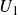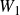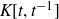Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Martinez Correa, Daniela
and
Koshlukov, Plamen
2023.
Specht property of varieties of graded Lie algebras.
Monatshefte für Mathematik,
Vol. 202,
Issue. 1,
p.
65.
FIDELIS, CLAUDEMIR
and
KOSHLUKOV, PLAMEN
2023.
-graded identities of the Lie algebras in characteristic 2.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 174,
Issue. 1,
p.
49.
Fidelis, Claudemir
Guimarães, Alan
and
Koshlukov, Plamen
2023.
A note on -gradings on the Grassmann algebra and elementary number theory.
Linear and Multilinear Algebra,
Vol. 71,
Issue. 7,
p.
1244.
Fidelis, Claudemir
Diniz, Diogo
and
Koshlukov, Plamen
2024.
Z-graded identities of the Virasoro algebra.
Journal of Algebra,
Vol. 640,
Issue. ,
p.
401.
Garcia, Micael Said
and
Yasumura, Felipe Yukihide
2025.
Graded polynomial identities of the infinite-dimensional upper triangular matrices over an arbitrary field.
Journal of Algebra,
Vol. 667,
Issue. ,
p.
778.
Fideles, Claudemir
and
Koshlukov, Plamen
2025.
Recent Progress in Ring and Factorization Theory.
Vol. 477,
Issue. ,
p.
205.