Moduli spaces of algebraic curves with rational maps
Published online by Cambridge University Press: 24 October 2008
Extract
Let ℳg be the coarse moduli scheme of curves of genus g. For an algebraically closed field k define 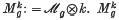 is a quasiprojective algebraic variety over k, its dimension being 3g – 3 for g ≥ 2, 1 for g = 1, and 0 for g = 0. It can be considered as the moduli variety for the classes of birationally equivalent curves of genus g over k. For 0 < g, g′ and n ≥ 1 let
is a quasiprojective algebraic variety over k, its dimension being 3g – 3 for g ≥ 2, 1 for g = 1, and 0 for g = 0. It can be considered as the moduli variety for the classes of birationally equivalent curves of genus g over k. For 0 < g, g′ and n ≥ 1 let 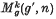 be the subset of those points of
be the subset of those points of  whose corresponding curves possess a rational map of degree n into a curve of genus g′ over k.
whose corresponding curves possess a rational map of degree n into a curve of genus g′ over k.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 78 , Issue 2 , September 1975 , pp. 283 - 292
- Copyright
- Copyright © Cambridge Philosophical Society 1975
References
REFERENCES
- 7
- Cited by


