Non-singular models of specialized Weddle surfaces
Published online by Cambridge University Press: 24 October 2008
Extract
There are, in projective space Σ of three dimensions, two famous quartic surfaces: W, the Weddle surface with six nodes ((13), p. 69, footnote) and K, the Kummer surface with sixteen ((s), p. 246, (7) passim). They are in birational correspondence and have the same non-singular model: the octavic base surface F of the net of quadrics in [5], which contain a given line λ and for which a given simplex S is self-polar ((5); (6)). One naturally takes S, with vertices X0, X1, X2, X3, X4, X5 as simplex of reference for homogeneous coordinates x0, x1, x2, x3, x4, x5; F is invariant under the harmonic inversions hj in the vertices Xj and opposite bounding primes xj = 0 of S. These six hj, mutually commutative and having identity for their product, generate an elementary abelian group 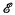 of order 32. This representation of
of order 32. This representation of 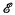 throws into prominence what may, in this context, be called its positive subgroup
throws into prominence what may, in this context, be called its positive subgroup 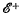 , of order 16, consisting of identity and the 15 products hjhk = hkhj; these are harmonic inversions in the edges XjXk and opposite bounding solids xj = xk = 0 of S. The coset of
, of order 16, consisting of identity and the 15 products hjhk = hkhj; these are harmonic inversions in the edges XjXk and opposite bounding solids xj = xk = 0 of S. The coset of 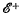 consists of the six hj and their ten products in threes, complementary products being the same (h0h1h2 ≡ h3h4h5) because of the product of all six hj being identity. These ten products are harmonic inversions in the ten pairs of opposite plane faces of S.
consists of the six hj and their ten products in threes, complementary products being the same (h0h1h2 ≡ h3h4h5) because of the product of all six hj being identity. These ten products are harmonic inversions in the ten pairs of opposite plane faces of S.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 80 , Issue 3 , November 1976 , pp. 399 - 418
- Copyright
- Copyright © Cambridge Philosophical Society 1976
References
REFERENCES
- 3
- Cited by


