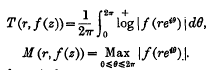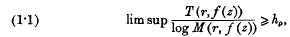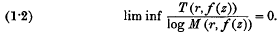Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Anderson, J. M.
1966.
Regularity criteria for integral and meromorphic functions.
Transactions of the American Mathematical Society,
Vol. 124,
Issue. 2,
p.
185.
Fuchs, W. H. J.
1967.
Developments in the classical Nevanlinna theory of meromorphic functions.
Bulletin of the American Mathematical Society,
Vol. 73,
Issue. 3,
p.
275.
Tsuzuki, Masanobu
and
Misu, Takehiro
1969.
A remark on a conjecture of paley.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 45,
Issue. 6,
Toda, Nobushige
1969.
Comparison between $T(r,f)$ and ${\rm log}M(r,f)$.
Tohoku Mathematical Journal,
Vol. 21,
Issue. 2,
Govorov, N. V.
1969.
Paley's hypothesis.
Functional Analysis and Its Applications,
Vol. 3,
Issue. 2,
p.
115.
Toda, Nobushige
1969.
Sur quelques fonctions entières ou méromorphes à l'égard de la conjecture de Paley.
Tohoku Mathematical Journal,
Vol. 21,
Issue. 4,
Niinō, Kiyoshi
1973.
On the growth of algebroid functions of finite lower order.
Kodai Mathematical Journal,
Vol. 25,
Issue. 4,
Fridman, A. N.
1981.
Lower bounds for subharmonic functions.
Ukrainian Mathematical Journal,
Vol. 32,
Issue. 5,
p.
472.
Sodin, M. L.
1981.
Relation between the sets of defect values and deviations for a meromorphic function of a finite order.
Siberian Mathematical Journal,
Vol. 22,
Issue. 2,
p.
320.
1989.
Subharmonic Functions.
p.
865.
Gol'dberg, A. A.
and
Ostrovskii, I. V.
1990.
Paley effect for entire characteristic functions and entire functions represented by dirichlet series.
Journal of Soviet Mathematics,
Vol. 48,
Issue. 3,
p.
255.
Vasyl’kiv, Ya. V.
Kondratyuk, A. A.
and
Tarasyuk, S. I.
1996.
General páley problem.
Ukrainian Mathematical Journal,
Vol. 48,
Issue. 1,
p.
27.
Kaluzhinova, L. V.
and
Marchenko, I. I.
2009.
On the magnitudes of deviations of entire functions of infinite order from rational functions.
Mathematical Notes,
Vol. 85,
Issue. 1-2,
p.
20.
Калужинова, Людмила Васильевна
Kaluzhynova, Lyudmyla Vasilevna
Марченко, Иван Иванович
and
Marchenko, Ivan Ivanovich
2009.
О величинах отклонений целых функций бесконечного порядка от функций рациональных.
Математические заметки,
Vol. 85,
Issue. 1,
p.
22.
Kowalski, Arnold
and
Marchenko, Ivan I.
2024.
On Defects of Entire Curves of Finite Lower Order.
Taiwanese Journal of Mathematics,
Vol. 28,
Issue. 6,
Kowalski, Arnold
and
Marchenko, Ivan I.
2025.
On Baernstein’s theorem for minimal surfaces.
Analysis,
Vol. 45,
Issue. 3,
p.
171.