Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-14T22:32:00.246Z Has data issue: false hasContentIssue false
  • English
  • Français

Note on the multiplication of series by Cauchy's rule

Published online by Cambridge University Press:  24 October 2008

G. H. Hardy
G. H. Hardy
Affiliation:
Trinity CollegeCambridge
Get access Rights & Permissions [Opens in a new window]

Extract

1. I proved in 1908(1) that if A = Σam and B = Σbn are convergent, and

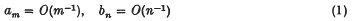
for large m and n, then

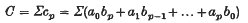
is convergent (necessarily to AB); and this theorem has been extended in a number of directions both by other writers and by myself. Thus we may replace (1), when am and bn are real, by

we may use conditions unsymmetrical in am and bn; we may put the same problem for the product of any number of series; and we may consider modes of ‘Dirichlet multiplication’ based on sequences λm and μn, reducing to Cauchy's when λm = m and μn = n. The appropriate references will be found in(2).

Type
Research Notes
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 40 , Issue 3 , November 1944 , pp. 251 - 252
Copyright
Copyright © Cambridge Philosophical Society 1944

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Hardy, G. H.Proc. London Math. Soc. (2), 6 (1908), 410.CrossRefGoogle Scholar
(2)Hardy, G. H.Journal London Math. Soc., 2 (1927), 169.CrossRefGoogle Scholar
(3)Neder, L.Proc. London Math. Soc. (2), 23 (1924), 176.Google Scholar