On radial basis approximation on periodic grids
Published online by Cambridge University Press: 24 October 2008
Extract
A radial basis function approximation in n variables has the form
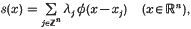
where ø:ℝn → ℝ denotes the n-variate, spherically symmetric function associated with a prescribed radial basis function ø+:ℝ+ → ℝ, i.e. ø = ø+(‖ · ‖), the norm being Euclidean. The 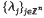 are real coefficients (often, approximants s above are considered where only finitely many λjs are non-zero), and
are real coefficients (often, approximants s above are considered where only finitely many λjs are non-zero), and 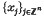 is a fixed set of points in ℝn (of course, only the xj with non-zero coefficient λj affect s). Thus s is a linear combination of translates of a radially symmetric function which can be of global support, the simplest choice being
is a fixed set of points in ℝn (of course, only the xj with non-zero coefficient λj affect s). Thus s is a linear combination of translates of a radially symmetric function which can be of global support, the simplest choice being 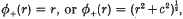 , where c is a positive parameter. The latter is referred to as the multiquadric function and is usefull in applications.
, where c is a positive parameter. The latter is referred to as the multiquadric function and is usefull in applications.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 112 , Issue 2 , September 1992 , pp. 317 - 334
- Copyright
- Copyright © Cambridge Philosophical Society 1992
References
REFERENCES
- 6
- Cited by


